-
One of the main aims of nuclear and particle physics communities is to understand hadrons in terms of the elementary excitations of quantum chromo-dynamics (QCD), which are quarks and gluons (the interested reader is referred to the Particle Data Group (PDG) and its topical mini-reviews [1]). QCD is well understood in its high energy regime as perturbative theoretical calculations have been contrasted with many experimental results since the inception of QCD 50 years ago. However, hadrons exist in a non-perturbative regime where, a priori, low-level rules produce high-level phenomena with significant apparent complexity [2]. In other words, for instance, less than 2% of a nucleon's mass can be attributed to the so-called current-quark masses that appear in the QCD Lagrangian, a phenomenon known as dynamical chiral symmetry breaking (DCSB). Another important non-perturbative effect is color confinement, which basically states that quarks and gluons (color objects) are not degrees of freedom readily accessible via experiment, i.e., they are confined inside hadrons.
This complexity makes hadron spectroscopy, the collection of readily accessible states constituted from gluons and quarks, the starting point for all further investigations. A very successful classification scheme for hadrons in terms of their valence quarks and antiquarks is the so-called quark model [3, 4], which basically separates hadrons in quark-antiquark (meson) and three-quark (baryon) configurations. The quark model, and its more modern variations and extensions, have been experimentally verified since the late 1960s and, with some caveats, they have been demonstrated to be very valuable. For instance, the phenomenological quark models represent a reliable theoretical approach to hadron spectra in heavy quark sectors, are sufficiently flexible to apply to exotic matter, and enable the easy computation of electromagnetic, weak, and strong reactions, whose predictions have been very useful in experimental searches.
Among the wide range of chiral quark models developed over the last 50 years [5], our theoretical framework is a QCD-inspired constituent quark model (CQM) proposed in Ref. [6] and extensively reviewed in Refs. [7, 8]. Moreover, the CQM has been recently applied to conventional mesons containing heavy quarks, describing a wide range of physical observables that involve spectra [9, 10], strong decays [11, 12], hadronic transitions [13, 14], and electromagnetic and weak reactions [15, 16]. Moreover, the interested reader may appreciate that the naive model has been extended to describe meson-meson molecules [17] and compact multiquark systems [18].
To extend our CQM in the baryon sector, we must take three steps: (i) compute baryon spectra, (ii) model a baryon decaying strongly into a meson plus another baryon, and (iii) describe baryon-meson interactions and their resulting bound- and resonance-states from the quark–(anti-)quark forces dictated by CQM. All these are being undertaken (see, for example, the advances in the third case by one of us in Refs. [19, 20]). However, the first task that has been completed by our group is the extension of the phenomenological
$ {}^3P_0 $ model to describe baryon strong decays. The same decay model was used in [21] to calculate the total strong decay widths of mesons, which belong to heavy quark sectors. Therein, a global fit of the experimental data showed that, contrary to the usual wisdom, the only free parameter of the$ {}^3P_0 $ model depends on the meson sector; thus, the scale-dependent strength follows a logarithmic behavior with respect the typical scale of the particular meson sector (Eq. (10) in Ref. [21]).Hadron strong decay is a complex non-perturbative process that has not yet been described from the first principles of QCD. In the search for explainations, Micu [22] formulated the
$ ^3P_0 $ model in the 1960s to obtain hadron decay rates using the corresponding wave functions and a strength parameter as the only required inputs. His approach was innovative for its simplicity and the few assumptions that were made. A few years later, Le Yaouanc et al. [23] developed Micu's model using the work of Carlitz and Kislinger based on$ S U(6)_w $ theory [24]. The remarkable features of this research were the assumption that constituent quarks drive the decay process and the use of harmonic oscillator wave functions to determine analytic expressions of the terms fitted by Micu from experiments. The only free parameter was the so-called pair-creation constant, γ. The research of Le Yaouanc et al. facilitated the calculation of many ratios between decay widths of mesons and baryons [25], popularizing the model. In the following years, the$ ^3P_0 $ model was widely used to describe decay properties of hadrons, such as charmonium states [26, 27]. In 1982, Hayne et al. improved the analytic expression corresponding to the transition matrix [28]. In 1996, Blundell et al. analyzed the data of various decay widths to fit the strength parameter γ [29], finding a value that is frequently used in modern works such as [30], but other values can be possible [31−41] depending on the specific details. In addition, a parallel study on the flux-tube pair creation model [42] showed that it contains, and thus can be simplified to, the$ ^3P_0 $ model, making it even more famous. Recent variants of the$ ^{3}P_{0} $ model modify the pair production vertex [43] or modulate the spatial dependence of the pair-production amplitude [44].This study involves determining a detailed analytical expression of the transition matrix of a baryon decaying into a meson-baryon system using the
$ ^3P_0 $ strong decay model to parametrize the required quark-antiquark pair creation from a vacuum and Gaussian expansions of the hadron wave functions to simplify the evaluation of matrix elements and express them in terms of the measured hadron sizes. The strength γ of the decay interaction is fixed to our previous evaluation of meson strong decays [21] to provide a free-parameter prediction of the$ \Delta(1232)\to \pi N $ decay width. Comparing it with the experimental value enables us to assess our calculation and possibly extend our decay model from the meson sector to the baryon one.The remainder of this article is organized as follows. Sec. II provides a detailed description of the
$ ^3P_0 $ model and derivation of the transition matrix, starting from the initial and final hadron states and the transition operator. A few assumptions are made to simplify the expression, and their limitations are specified. Section III presents an application of the model, obtaining the decay width of the process$ \Delta(1232)\to \pi N $ , and the data used are specified. In this section, the quark-antiquark pair creation constant, γ, for baryons appears to follow the analytic expression presented in [21] for mesons. Finally, we summarize the study and draw some conclusions in Sec. IV. -
The quark-antiquark pair creation models are phenomenological models to describe hadron strong decays. Among these models, the
$ ^3P_0 $ strong decay model is the most popular. It states that the quark-antiquark pair created from a vacuum must conserve the vacuum's angular momentum, parity, and charge conjugation, viz. the quark-antiquark pair must have$ J^{PC} = 0^{++} $ quantum numbers. Another important property of the$ {}^3P_0 $ model is that it considers only diagrams in which the quark-antiquark pair separates into different final hadrons. This was originally motivated by experimental observations and it is known as the Okubo-Zweig-Iizuka (OZI) rule [45−47], which states that disconnected diagrams are more suppressed than connected ones.The model defined as above describes baryon into meson
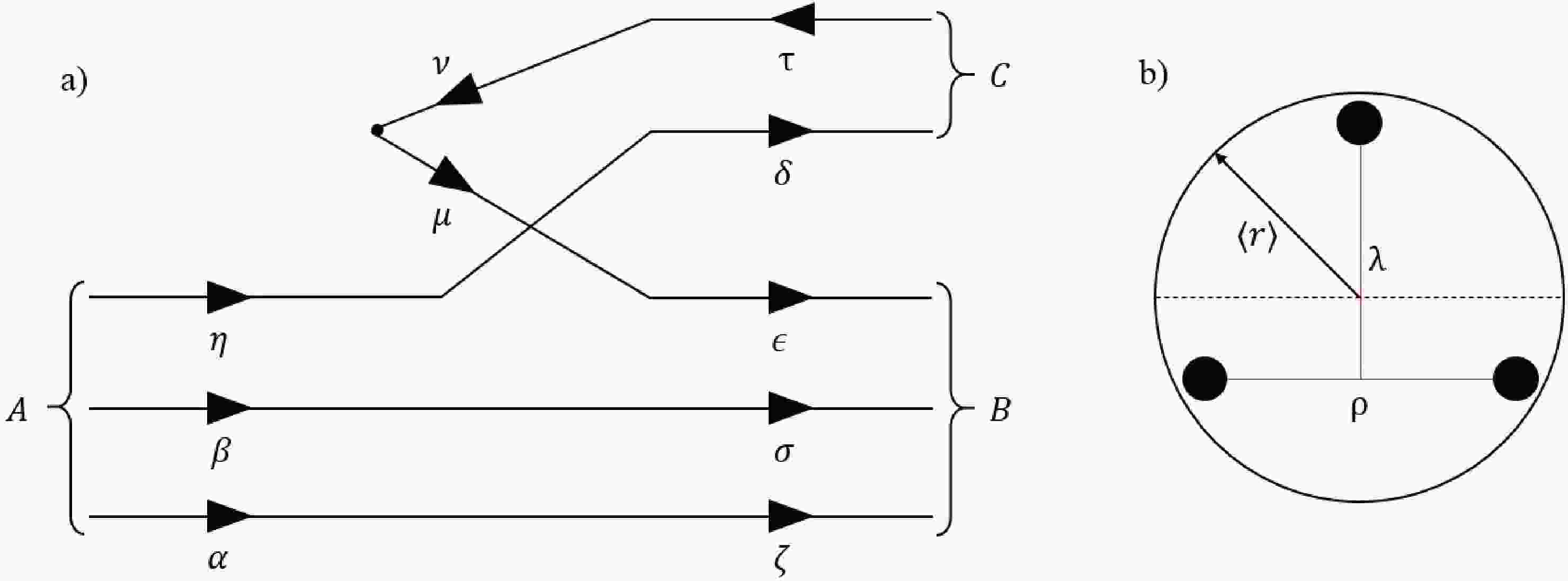
$ + $ baryon strong decays as represented in panel (a) of Fig. 1. Thus, its associated transition operator is given by
Figure 1. (a) Feynman diagram of a baryon, A, decaying into a baryon, B, and a meson, C. (b) Schematic representation of a baryon as an sphere of radius
$ \langle r \rangle$ ; a particular set of Jacobi coordinates,$ (\rho,\lambda)$ , for the quarks in a baryon is also shown.$ \begin{aligned}[b] T =\;& -3\gamma'\sum\limits_{\mu,\nu} \int {\rm d}^3p_\mu d^3p_\nu \left[ {\cal{Y}}_1\left( \frac{\vec{p}_\mu - \vec{p}_{\nu}}{2}\right) \otimes (s_\mu s_\nu)_1 \right]_0 \\ & \times a^{\dagger}_{\mu}(\vec{p}_\mu) b^{\dagger}_{\nu}(\vec{p}_\nu)\delta^{(3)}(\vec{p_\mu}+\vec{p_\nu}), \end{aligned} $

(1) where μ is the quark, and ν is the antiquark created. The
$ 3 $ -dimensional Dirac delta function,$ \delta^{(3)}(\vec{p_\mu}+\vec{p_\nu}) $ , ensures the conservation of momenta, and the function$ {\cal{Y}}_l(\vec{p}\,) = p^l\, Y_{l}(\hat{p}) $ is the solid harmonic that characterizes the angular momentum ($ l = 1 $ ) of the pair created. We can also observe that it is coupled to the spin-$ 1 $ of the pair to provide a total angular momentum of$ J = 0 $ . Meanwhile,$ \gamma' $ is the only unknown constant of the$ {}^3P_0 $ model that characterizes the strength of the quark-antiquark pair creation from the vacuum, and it is normally fitted to the data. Moreover, note that this transition operator is a non-relativistic reduction of an interacting Hamiltonian involving Dirac quark fields that describe the production process [21]. Here,$ \sqrt{3} $ is replaced by$ 3 $ when transitioning from meson decays to baryon ones because the term must cancel out with the color contribution.The decay width of the process can be calculated using the following relation:
$ \Gamma_{A \rightarrow BC} = 2\pi \frac{E_B(k_0)E_C(k_0)}{m_Ak_0}\sum\limits_{J_{BC},l}|{\cal{M}}_{A\rightarrow BC}|^2, $

(2) where
$ k_0 $ is the relative momentum of the final products with respect to the initial state [48]. The squared modulus of the invariant matrix element must be summed over all possible values of$ J_{BC} $ and the relative angular momentum l whose inner product is equal to the total angular momentum of the decaying baryon,$ J_A $ .To calculate the invariant matrix element that appears in the formula of the decay width,
$ {\cal{M}}_{A \rightarrow BC} = \delta^{(3)}(\vec{K}_0) \langle BC | T | A \rangle \,, $

(3) where
$ \vec{K}_0 $ is the center-of-mass momentum of the decaying baryon, we must establish expressions for the initial and final states:$ \begin{aligned}[b] |A\rangle =\;& \sum\limits_{M_{L_A},M_{S_A}} \langle L_A M_{L_A} S_A M_{S_A} | J_A M_{J_A}\rangle \\ \;& \times \int {\rm d}^3p_\alpha {\rm d}^3p_\beta {\rm d}^3p_\eta \delta^{(3)}(\vec{P}_A - \vec{K}_A)\chi_A {\cal C}_A\\ & \times {\cal I}_A \phi_A(\vec{p}_\alpha,\vec{p}_\beta,\vec{p}_\eta) a^\dagger_\alpha(\vec{p}_\alpha) a^\dagger_\beta(\vec{p}_\beta) a^\dagger_\eta(\vec{p}_\eta) |0\rangle \,, \end{aligned} $

(4) $ \begin{aligned} |BC\rangle =\;& \int {\rm d}^3K_B {\rm d}^3K_C \sum\limits_{m,M_B,M_C,M_{I_B},M_{I_C}} \langle J_{BC}M_{BC}lm|J_AM_A \rangle \delta^{(3)}(\vec{K}-\vec{K}_0)\delta(k-k_0) \frac{Y_{lm}(\hat{k})}{k} \langle J_BM_BJ_CM_C|J_{BC}M_{BC} \rangle \\ \;& \times\langle I_BM_{I_B}I_CM_{I_C}|I_{BC}M_{I_{BC}} \rangle \int {\rm d}^3p_\delta {\rm d}^3p_\epsilon {\rm d}^3p_\zeta d^3p_\sigma {\rm d}^3p_\tau \delta^{(3)}(\vec{K}_B - \vec{P}_B)\delta^{(3)}(\vec{K}_C - \vec{P}_C) \\ \;& \times \chi_B {\cal C}_B {\cal I}_B \phi_B(\vec{p}_\sigma,\vec{p}_\zeta,\vec{p}_\epsilon) a^\dagger_\sigma(\vec{p}_\sigma) a^\dagger_\zeta(\vec{p}_\zeta) a^\dagger_\epsilon(\vec{p}_\epsilon) \chi_C {\cal C}_C {\cal I}_C \phi_C(\vec{p}_\delta,\vec{p}_\tau) a^\dagger_\delta(\vec{p}_\delta) b^\dagger_\tau(\vec{p}_\tau) |0\rangle. \end{aligned} $

(5) In these equations, the functions
$ \phi_{A,B,C} $ are the Fourier transforms of the hadron’s wave functions in coordinate space. They describe the probability of finding the hadron in momentum space. Moreover,$ \chi_{A,B,C} $ ,$ {\cal C}_{A,B,C} $ , and$ {\cal I}_{A,B,C} $ are the spin, color, and isospin wave functions of the hadrons involved in the decay, respectively. In the final state$ |BC\rangle $ , the internal products ensure the conservation of angular momentum and isospin between the baryon and meson in the final state but also its coupling with the initial baryon state.The invariant matrix element,
$ {\cal M}_{A\to BC} $ , is a product of a color factor, a flavor factor, and a spin-space overlap integral, i.e.$ {\cal{M}}_{A\rightarrow BC} = {\cal{I}}_{\rm Color} \, {\cal{I}}_{\rm Flavor} \, {\cal{I}}_{\rm Spin-space} \,, $

(6) such that each component can be calculated separately.
-
Before discussing the spin-space contribution, we must determine how many equivalent Feynman diagrams contribute to the same process, i.e., the symmetry factor. Focusing on the ladder operators that appear in Eqs. (1), (4) and (5), where combined adequately, we arrive at the following expression:
$ \langle 0 | b_\tau(\vec{p}_\tau) a_\delta(\vec{p}_\delta) a_\epsilon(\vec{p}_\epsilon) a_\zeta(\vec{p}_\zeta) a_\sigma(\vec{p}_\sigma) a^\dagger_\mu(\vec{p}_\mu) b_\nu^\dagger(\vec{p}_\nu) a_\alpha^\dagger(\vec{p}_\alpha) a_\beta^\dagger(\vec{p}_\beta) a_\eta^\dagger(\vec{p}_\eta) |0 \rangle \,. $

(7) This product of creation and annihilation operators can be simplified. Because quarks are fermions, we use the anti-commutation relations of the ladder operators,
$ \{ a_r(\vec{p}\,),a^{\dagger}_s(\vec{p}\,') \} = a_r(\vec{p}\,)a^{\dagger}_s(\vec{p}\,')+a^{\dagger}_s(\vec{p}\,')a_r(\vec{p}\,) = \delta_{rs}\delta^{(3)}(\vec{p} - \vec{p}\,') \,, $

(8) $ \{ a_r(\vec{p}\,),b^{\dagger}_s(\vec{p}\,') \} = \{ a_r(\vec{p}\,),b_s(\vec{p}\,') \} = \{ a_r^{\dagger}(\vec{p}\,),b_s(\vec{p}\,') \} = \{ a_r^{\dagger}(\vec{p}\,),b^{\dagger}_s(\vec{p}\,') \} = 0 \,, $

(9) $ \{ a_r(\vec{p}\,),a_s(\vec{p}\,') \} = \{ a_r^{\dagger}(\vec{p}\,),a^{\dagger}_s(\vec{p}\,') \} = \{ b_r(\vec{p}\,),b_s(\vec{p}\,') \} = \{ b_r^{\dagger}(\vec{p}\,),b^{\dagger}_s(\vec{p}\,') \} = 0 \,, $

(10) and arrange them in normal ordering to arrive at
$ \begin{aligned}[b] \langle 0 | b_\tau a_\sigma a_\zeta a_\epsilon a_\delta a^\dagger_\mu b^\dagger_\nu a^\dagger_\alpha a^\dagger_\beta a^\dagger_\eta | 0 \rangle =\; & \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\epsilon\alpha} \delta_{\zeta\beta} \delta_{\sigma\eta} - \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\epsilon\alpha} \delta_{\sigma\beta} \delta_{\zeta\eta} - \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\zeta\alpha} \delta_{\epsilon\beta} \delta_{\sigma\eta} - \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\delta\alpha} \delta_{\zeta\beta}+ \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\zeta\alpha} \delta_{\sigma\beta} \delta_{\epsilon\eta} \delta_{\sigma\eta} \\ & + \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\sigma\alpha} \delta_{\epsilon\beta} \delta_{\zeta\eta} + \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\delta\alpha} \delta_{\sigma\beta} \delta_{\zeta\eta} + \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\zeta\alpha} \delta_{\delta\beta} \delta_{\sigma\eta} + \delta_{\tau\nu} \delta_{\zeta\mu} \delta_{\delta\alpha} \delta_{\epsilon\beta} \delta_{\sigma\eta} - \delta_{\tau\nu} \delta_{\delta\mu} \delta_{\sigma\alpha} \delta_{\zeta\beta} \delta_{\epsilon\eta}\\ & - \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\zeta\alpha} \delta_{\sigma\beta} \delta_{\delta\eta} - \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\sigma\alpha} \delta_{\delta\beta} \delta_{\zeta\eta} - \delta_{\tau\nu} \delta_{\zeta\mu} \delta_{\delta\alpha} \delta_{\sigma\beta} \delta_{\epsilon\eta} - \delta_{\tau\nu}\delta_{\zeta\mu} \delta_{\epsilon\alpha} \delta_{\delta\beta} \delta_{\sigma\eta} - \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\delta\alpha} \delta_{\epsilon\beta} \delta_{\zeta\eta}\\ & + \delta_{\tau\nu}\delta_{\epsilon \mu} \delta_{\sigma\alpha} \delta_{\zeta\beta} \delta_{\delta\eta} + \delta_{\tau\nu}\delta_{\zeta\mu} \delta_{\epsilon\alpha} \delta_{\sigma\beta} \delta_{\delta\eta} + \delta_{\tau\nu} \delta_{\zeta\mu} \delta_{\sigma\alpha} \delta_{\delta\beta} \delta_{\epsilon\eta} + \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\delta\alpha} \delta_{\zeta\beta} \delta_{\epsilon\eta} + \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\epsilon\alpha} \delta_{\delta\beta} \delta_{\zeta\eta} \\ & - \delta_{\tau\nu} \delta_{\zeta\mu} \delta_{\sigma\alpha} \delta_{\epsilon\beta} \delta_{\delta\eta} - \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\epsilon\alpha} \delta_{\zeta\beta} \delta_{\delta\eta} - \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\zeta\alpha} \delta_{\delta\beta} \delta_{\epsilon\eta} + \delta_{\tau\nu} \delta_{\sigma\mu} \delta_{\zeta\alpha} \delta_{\epsilon\beta} \delta_{\delta\eta}. \end{aligned}$

(11) Note here that we have abused the notation,
$ \delta_{ab}\,\delta^{(3)}(\vec{p}_a-\vec{p}_b) \equiv \delta_{ab} $ .Each term is a different process that can be represented by a Feynman diagram. Following the OZI rule, terms with the factor
$ \delta_{\delta\mu} $ can be eliminated as the created quark and antiquark combine into the same final hadron; the remaining ones are pictorially shown in Fig. 2. If all quarks and antiquarks involved in the baryon strong decay are indistinguishable, as in the case of$ \Delta\to \pi N $ , the diagrams can be considerd to be equivalent. Therefore, the final result may be expressed as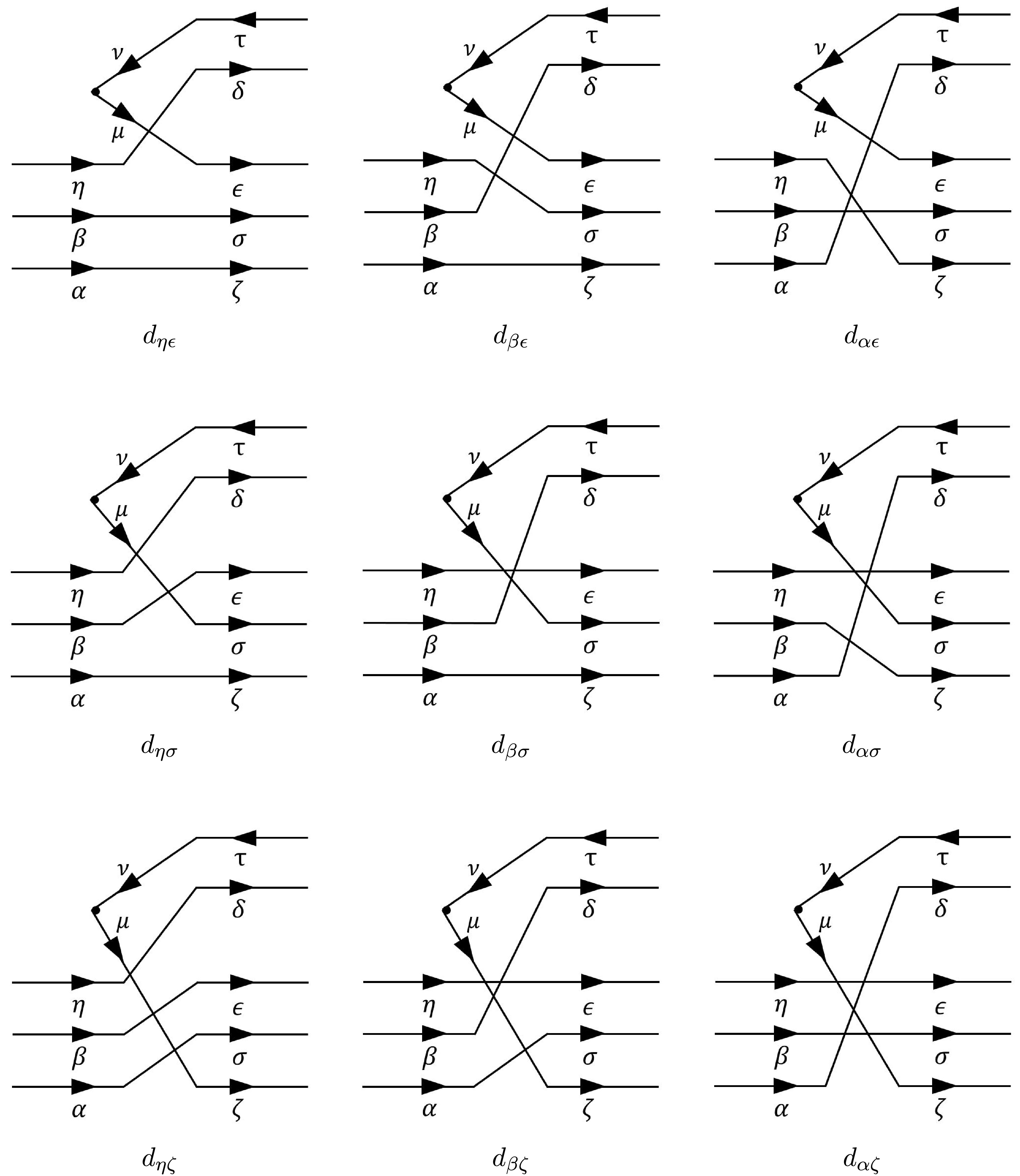
Figure 2. Decay process for a baryon. Below each diagram, the notation
$ d_{ab}$ characterizes the diagram using the quark$ (a)$ that begins in the baryon and ends in the meson and the quark$ (b)$ that results from the pair created and ends in the baryon.$ \langle 0|b_\tau a_\sigma a_\zeta a_\epsilon a_\delta a^\dagger_\mu b^\dagger_\nu a^\dagger_\alpha a^\dagger_\beta a^\dagger_\eta|0 \rangle = - 18 \, \delta_{\tau\nu} \delta_{\epsilon\mu} \delta_{\zeta\alpha} \delta_{\sigma\beta} \delta_{\delta\eta} \,. $

(12) If this equivalence between quarks and antiquarks does not hold, the contribution of different diagrams differs between them but does not significantly change the subsequent expressions. Thus, we may straightforwardly extend the computation to describe these cases.
Now, the spin-space contribution can be separated in two terms: one collects the coupling of angular momentum and spin,
$ ({\cal{J}}) $ , and the other involves linear momenta,$ ({\cal{E}}) $ ,$ {\cal{I}}_{\rm Spin-space} = 54\gamma' \sum\limits_{L_{BC},L,S} {\cal{J}}(A\rightarrow BC) {\cal{E}}(A\rightarrow BC) \,. $

(13) The total angular momentum of the hadron, J, represents a coupling between its angular momentum, L, and spin, S. Therefore, the initial form of
$ {\cal{J}}(A\rightarrow BC) $ is$ \begin{aligned}[b] {\cal{J}}(A\rightarrow BC) =\;& \left\{[L_BS_B]_{J_B} [L_CS_C]_{J_C} \right\}_{J_{BC}}^* \{[L_{BC}S]_{J_{BC}} \, l\}_{J_A}^* \\ & \times \{[L_AS_A]_{J_A} [11]_0\}_{J_A} \,, \end{aligned} $

(14) where the extra
$ [11]_0 $ is included to consider the quantum numbers of the quark-antiquark pair created from the vacuum. Note also that complex conjugate symbols affect to final states as expected.Equation (14) is a matrix element expressed in terms of hadron’s individual
$ L-S $ coupling into J. The final expression must have a total angular momentum$ (L) $ , which is the inner sum of all the angular momenta of the particles, and a total spin$ (S) $ , which is the inner sum of all the spins of the particles. These final angular momentum and spin must be then coupled to the total angular momentum of the decaying baryon. These transformations can be performed using Wigner symbols [49]:$ \begin{aligned}[b] {\{ {[{L_1}{S_1}]_{{J_1}}},{[{L_2}{S_2}]_{{J_2}}}\} _{{J_T}}} =\;& \sum\limits_{{L_T},{S_T}} {{\Pi _{{J_1},{J_2},{L_T},{S_T}}}} \left\{ {\begin{array}{*{20}{c}} {{L_1}}&{{S_1}}&{{J_1}}\\ {{L_2}}&{{S_2}}&{{J_2}}\\ {{L_T}}&{{S_T}}&{{J_T}} \end{array}} \right\} \\ &\times \{ {[{L_1}{L_2}]_{{L_T}}},{[{S_1}{S_2}]_{{S_T}}}]{\} _{{J_T}}}, \end{aligned} $

(15) where
$ \Pi_l = \sqrt{2l+1} $ is used to simplify the notation. With this relation, the couplings of the initial state and the pair created can be changed as$ \begin{aligned}[b] {\{ {[{L_A}{S_A}]_{{J_A}}}{[11]_0}\} _{{J_A}}} =\;& \sum\limits_{L,S} {{\Pi _{L,S,{J_A},0}}} \left\{ {\begin{array}{*{20}{c}} {{L_A}}&{{S_A}}&{{J_A}}\\ 1&1&0\\ L&S&{{J_A}} \end{array}} \right\}\\ & \times{\left\{ {{{[{L_A}1]}_L}{{[{S_A}1]}_S}} \right\}_{{J_A}}}{\mkern 1mu} . \end{aligned} $

(16) Because the
$ 9J $ -symbol contains a zero in one of its components, it can be reduced to a$ 6J $ -symbol [49]:$ \begin{aligned}[b] {\left\{ {{{[{L_A}{S_A}]}_{{J_A}}}{{[11]}_0}} \right\}_{{J_A}}} =\;& \sum\limits_{L,S} {{{( - 1)}^{S + {J_A} + {L_A} + 1}}} \frac{{{\Pi _{L,S}}}}{{\sqrt 3 }}\\ & \times\left\{ {\begin{array}{*{20}{c}} L&S&{{J_A}}\\ {{S_A}}&{{L_A}}&1 \end{array}} \right\}{\left\{ {{{[{L_A}1]}_L}{{[{S_A}1]}_S}} \right\}_{{J_A}}}. \end{aligned} $

(17) A similar transformation can be performed for the final state:
$ \left\{ {{{[{L_B}{S_B}]}_{{J_B}}}{{[{L_C}{S_C}]}_{{J_C}}}} \right\}_{{J_{BC}}}^* = \sum\limits_{{L_{BC}},S} {{\Pi _{{L_{BC}},S,{J_B},{J_C}}}} \left\{ {\begin{array}{*{20}{c}} {{L_B}}&{{S_B}}&{{J_B}}\\ {{L_C}}&{{S_C}}&{{J_C}}\\ {{L_{BC}}}&S&{{J_{BC}}} \end{array}} \right\} \left\{ {{{[{L_B}{L_C}]}_{{L_{BC}}}}{{[{S_B}{S_C}]}_S}} \right\}_{{J_A}}^*, $

(18) where the conservation of spin is used, simplifying
$ S_{BC} = S $ . The baryon and meson in the final state have a relative angular momentum between them, denoted by l; reordering the terms as indicated in [49], we obtain$ \begin{aligned}[b] \{ {[{L_{BC}}S]_{{J_{BC}}}}{\mkern 1mu} l\} _{{J_A}}^* =\;& {( - 1)^{{L_{BC}} + S - {J_{BC}}}}\{ {[S{L_{BC}}]_{{J_{BC}}}}{\mkern 1mu} l\} _{{J_A}}^*\\ =\;& {( - 1)^{{L_{BC}} + S - {J_{BC}}}}\displaystyle\sum\limits_L {{{( - 1)}^{{L_{BC}} + S + {J_A} + l}}} {\Pi _{L,{J_{BC}}}}\left\{ {\begin{array}{*{20}{c}} S&{{L_{BC}}}&{{J_{BC}}}\\ l&{{J_A}}&L \end{array}} \right\}\{ S{[{L_{BC}}{\mkern 1mu} l]_L}\} _{{J_A}}^*\\ =\;& \displaystyle\sum\limits_L {{{( - 1)}^{2{L_{BC}} + 2S + {J_A} + l - {J_{BC}}}}} {\Pi _{L,{J_{BC}}}}\left\{ {\begin{array}{*{20}{c}} S&{{L_{BC}}}&{{J_{BC}}}\\ l&{{J_A}}&L \end{array}} \right\}{( - 1)^{S + L - {J_A}}}\{ {[{L_{BC}}{\mkern 1mu} l]_L}S\} _{{J_A}}^*\\ =\;& \displaystyle\sum\limits_L {{{( - 1)}^{S + L + l - {J_{BC}}}}} {\Pi _{L,{J_{BC}}}}\left\{ {\begin{array}{*{20}{c}} S&{{L_{BC}}}&{{J_{BC}}}\\ l&{{J_A}}&L \end{array}} \right\}\{ {[{L_{BC}}{\mkern 1mu} l]_L}S\} _{{J_A}}^*{\mkern 1mu} . \end{aligned}$

(19) The spin couplings can be also simplified. The corresponding matrix element,
$ \{[s_\mu s_\beta s_\alpha]_{S_B}[s_\nu s_\eta]_{S_C}\}_S^* \{[s_\alpha s_\beta s_\eta]_{S_A}[s_\mu s_\nu]_{1}\}_S \,, $

(20) has been written to consider the delta-functions of Eq. (12). Now, because the couplings are binary operations, the spins of the quarks inside baryons must be ordered. Using the Jacobi coordinate system
$ (\rho,\lambda) $ shown in panel (b) of Fig. 2, the named ρ-spin can be introduced$ s_\rho = s_\alpha \otimes s_\beta \,, $

(21) as the spin of the non-interacting quarks during the decay, i.e., quarks that do not change their properties in the process. Subsequently, the spin of the baryons becomes
$ \begin{array}{l} \left[ s_\alpha s_\beta s_\eta \right]_{S_A} = \left[s_\rho s_\eta \right]_{S_A} \,, \\ \left[ s_\mu s_\beta s_\alpha \right]_{S_B} = \left[ s_\mu \left( s_\beta s_\alpha \right)_{s_\rho} \right]_{S_B} = (-1)^{s_\alpha + s_\beta + s_\mu - S_B} \left[ s_\rho s_\mu \right]_{S_B} \,, \end{array}$

(22) and the spin conservation can be expressed using a
$ 9J $ -symbol:$ \begin{aligned}[b] & \{[s_\mu s_\beta s_\alpha]_{S_B} [s_\nu s_\eta]_{S_C}\}_S^* \{[s_\alpha s_\beta s_\eta]_{S_A}[s_\mu s_\nu]_{1}\}_S \\ =\;& {( - 1)^{{s_\alpha } + {s_\beta } + {s_\mu } + {s_\nu } + {s_\eta } - {S_B} - {S_C}}}{\Pi _{{S_B},{S_C},{S_A},1}}\left\{ {\begin{array}{*{20}{c}} {{s_\rho }}&{{s_\mu }}&{{S_B}}\\ {{s_\eta }}&{{s_\nu }}&{{S_C}}\\ {{S_A}}&1&S \end{array}} \right\}. \end{aligned} $

(23) When all couplings are modified, the final expression for
$ {\cal{J}}(A\rightarrow BC) $ becomes$ \begin{aligned}[b] {\cal{J}}(A\rightarrow BC) =\;& (-1)^{3/2 - S_B - S_C + L_A + L + l + J_A - J_{BC}} \, \Pi_{L_{BC},L,L,J_B,J_C,J_{BC},S_A,S_B,S_C,S,S} \times \left\{ {\begin{array}{*{20}{c}} L&S&{{J_A}}\\ {{S_A}}&{{L_A}}&1 \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} S&{{L_{BC}}}&{{J_{BC}}}\\ l&{{J_A}}&L \end{array}} \right\}\\ \;&\times \left\{ {\begin{array}{*{20}{c}} {{s_\rho }}&{1/2}&{{S_B}}\\ {1/2}&{1/2}&{{S_C}}\\ {{S_A}}&1&S \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{L_B}}&{{S_B}}&{{J_B}}\\ {{L_C}}&{{S_C}}&{{J_C}}\\ {{L_{BC}}}&S&{{J_{BC}}} \end{array}} \right\}. \end{aligned}$

(24) The remaining term to be calculated is the linear momentum contribution, whose initial expression is
$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) =\;& \int {\rm d}^3K_B {\rm d}^3K_C {\rm d}^3p_\alpha {\rm d}^3 p_\beta {\rm d}^3p_\eta {\rm d}^3p_\mu {\rm d}^3p_\nu \\ \;&\times \delta^{(3)}(\vec{K} - \vec{K}_0) \delta^{(3)}(\vec{K}_A - \vec{P}_A) \delta^{(3)}(\vec{K}_B - \vec{P}_B) \delta^{(3)}(\vec{K}_C - \vec{P}_C) \delta^{(3)}(\vec{p}_\mu + \vec{p}_\nu) \frac{\delta(k-k_0)}{k} \\ \;&\times \left\{ \left[ \phi_B(\vec{p}_\sigma,\vec{p}_\zeta,\vec{p}_\epsilon) \phi_C(\vec{p}_\delta,\vec{p}_\tau) \right]_{L_{BC}} Y_l(\hat{k}) \right\}_L^* \left\{ \phi_A(\vec{p}_\alpha,\vec{p}_\beta,\vec{p}_\eta) {\cal{Y}}_1 \left( \frac{\vec{p}_\mu - \vec{p}_\nu}{2} \right) \right\}_L \,. \end{aligned}$

(25) This expression can be simplified by defining a new set of coordinates:
$ \begin{array}{ll} \vec{P_A} = \vec{p}_\alpha + \vec{p}_\beta + \vec{p}_\eta \,, & \vec{P}_C = \vec{p}_\delta + \vec{p}_\tau \,, \\ \vec{p}_{\rho_A} = \dfrac{\omega_\beta \vec{p}_\alpha - \omega_\alpha \vec{p}_\beta}{\omega_{\alpha\beta}} \,, & \vec{p}_C = \dfrac{\omega_\delta\vec{p}_\tau - \omega_\tau \vec{p}_\delta}{\omega_{\delta\tau}} \,, \\ \vec{p}_{\lambda_A} = \dfrac{\omega_\eta(\vec{p}_\alpha + \vec{p}_\beta)- \omega_{\alpha\beta}\vec{p}_\eta}{\omega_{\alpha\beta\eta}} \,, & \vec{P} = \vec{p}_\mu + \vec{p}_\nu \,, \\ \vec{P_B} = \vec{p}_\zeta + \vec{p}_\sigma + \vec{p}_\epsilon \,, &\vec{p} = \dfrac{\vec{p}_\mu - \vec{p}_\nu}{2} \,, \\ \vec{p}_{\rho_B} = \dfrac{\omega_\sigma \vec{p}_\zeta - \omega_\zeta \vec{p}_\sigma}{\omega_{\zeta\sigma}} \,, & \vec{K} = \vec{K}_B + \vec{K}_C \,, \\ \vec{p}_{\lambda_B} = \dfrac{\omega_\epsilon(\vec{p}_\zeta + \vec{p}_\sigma)- \omega_{\zeta\sigma}\vec{p}_\epsilon}{\omega_{\zeta\sigma\epsilon}} \,, & \vec{k} = \dfrac{\omega_C\vec{K}_B - \omega_B\vec{K}_C}{\omega_{BC}} \,, \end{array} $

(26) where two relative momenta must be defined for baryons, whereas only one relative momentum is sufficient for mesons. Additionally, we introduce a so-called reduced mass convention that redefines all masses in terms of a reference one, m:
$ {{\omega _\alpha } = \frac{{{m_\alpha }}}{m}{\mkern 1mu} ,} {\quad \quad } {{\omega _{\alpha \beta }} = {\omega _\alpha } + {\omega _\beta }{\mkern 1mu} .} $

(27) The delta functions related to momenta provide an additional set of conditions,
$ \begin{aligned}[b] & \vec{K} = \vec{K}_0 = \vec{K}_A = \vec{P}_A = 0 \,,\\ & \vec{K}_B = \vec{P}_B \,,\\ & \vec{K}_C = \vec{P}_C \,,\\ & \vec{p}_\mu + \vec{p}_\nu = \vec{P} = 0 \,, \end{aligned} $

(28) where the center-of-mass of baryon A is taken as the center of mass of the interaction. Now, the equivalences in momenta eliminate some integrals, and the reaming variables can be expressed in terms of the following ones:
$ \begin{aligned}[b] & \vec{p} = \vec{p}_\mu = -\vec{p}_\nu \,,\\ & \vec{k} = \vec{p}_{\lambda_A} + \vec{p} \,,\\ & \vec{p}_\rho = \vec{p}_{\rho_A} = \vec{p}_{\rho_B} \,. \end{aligned} $

(29) Subsequently, the simplified expression for
$ {\cal{E}}(A \rightarrow BC) $ is$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) = \;&\int {\rm d}^3p {\rm d}^3k {\rm d}^3p_\rho\frac{\delta(k-k_0)}{k^{l+1}} \\ \;&\times \left\{ \left[ \phi_B(\vec{p}_\sigma,\vec{p}_\zeta,\vec{p}_\epsilon) \phi_C(\vec{p}_\delta,\vec{p}_\tau) \right]_{L_{BC}} {\cal{Y}}_l(\hat{k}) \right\}_L^* \\ & \times\left\{ \phi_A(\vec{p}_\alpha,\vec{p}_\beta,\vec{p}_\eta) {\cal{Y}}_1 \left( \frac{\vec{p}_\mu - \vec{p}_\nu}{2} \right) \right\}_L. \end{aligned}$

(30) Continuing with the calculation, the hadron wave functions can be separated in radial and angular parts:
$ \phi_A(\vec{p}_\alpha,\vec{p}_\beta,\vec{p}_\eta) = f_{\lambda_A}(\vec{p}_{\lambda_A}) f_{\rho}(\vec{p}_{\rho}) \left[{\cal{Y}}_{L_{\lambda_A}}(\vec{p}_{\lambda_A}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_A} \,, $

(31) $ \phi_B(\vec{p}_\sigma,\vec{p}_\zeta,\vec{p}_\epsilon) = f_{\lambda_B}(\vec{p}_{\lambda_B}) f_{\rho}(\vec{p}_{\rho}) \left[{\cal{Y}}_{L_{\lambda_B}}(\vec{p}_{\lambda_B}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_B} \,, $

(32) $ \phi_C(\vec{p}_C) = f_C(\vec{p}_C){\cal{Y}}_{L_C}(\vec{p}_C) \,, $

(33) where the solid spherical harmonics consider the Jacobi coordinate decomposition of a baryon system, and the radial parts are assumed to be Gaussian functions:
$ f_{\lambda_A}(\vec{p}_{\lambda_A}) = \sum\limits_i d_i^{\lambda_A} \exp\left( -\frac{{\lambda_A}_i}{2} {p}_{\lambda_A}^2 \right) \,, $

(34) $ f_{\lambda_B}(\vec{p}_{\lambda_B}) = \sum\limits_j d_j^{\lambda_B} \exp\left( -\frac{{\lambda_B}_j}{2} {p}_{\lambda_B}^2 \right) \,, $

(35) $ f_\rho(\vec{p}_\rho) = \sum\limits_k d_k^\rho \exp\left( -\frac{\rho_k}{2} {p}_\rho^2 \right) \,, $

(36) $ f_C(\vec{p}_C) = \sum\limits_{l'} d_{l'}^C \exp\left( -\frac{C_{l'}}{2} {p}_C^2 \right) \,, $

(37) where the constants can be computed theoretically from hadron spectra or fitted to experimental data of hadron radii.
1 The limits of the sums are fixed according to the precision required. Inserting the above expressions in Eq. (30), we obtain$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) =\;& \sum\limits_{ijkl'} d_i^{\lambda_A} d_j^{\lambda_B} (d_k^\rho)^2 d_{l'}^C \times \int {\rm d}^3p {\rm d}^3k {\rm d}^3p_{\rho} \frac{\delta(k-k_0)}{k^{l+1}} \exp\left( -\frac{1}{2} [{\lambda_A}_i p_{\lambda_A}^2 + {\lambda_B}_j p_{\lambda_B}^2 + \rho_k p_\rho^2 + C_{l'} p_C^2] \right) \\ \;&\times \left\{ \left[ \left[{\cal{Y}}_{L_{\lambda_B}}(\vec{p}_{\lambda_B}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_B} {\cal{Y}}_{L_C}(\vec{p}_C) \right]_{L_{BC}} {\cal{Y}}_l(\vec{k}) \right\}_L^* \left\{ \left[{\cal{Y}}_{L_{\lambda_A}}(\vec{p}_{\lambda_A}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_A} {\cal{Y}}_1 \left( \vec{p}\,\right) \right\}_L \,. \end{aligned} $

(38) We now need all functions of Eq. (38) to be expressed in terms of the integration variables. Hence, we define
$ \vec{q} = \vec{p} - x\vec{k} $ , where x can be any number; note also that p and$ p_\rho $ continue to be variables of the integral. Therefore,$ \begin{aligned}[b] & \vec{p}_{\lambda_A} = \left(1-x\right)\vec{k} - \vec{q} \,, \\ & \vec{p}_{\lambda_B} = \left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right)\vec{k} - \vec{q} \,, \\ & \vec{p}_C = \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right) \vec{k} - \vec{q} \,. \end{aligned} $

(39) The terms are squared,
$ \begin{aligned}[b] \;&p_{\lambda_A}^2 = \left(1-x\right)^2k^2 + q^2 - 2\left(1-x\right) \vec{k}\cdot\vec{q} \,, \\ \;&p_{\lambda_B}^2 = \left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right)^2k^2 + q^2 - 2\left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right)\vec{k}\cdot\vec{q} \,, \\ \;&p_C^2 = \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right)^2 k^2 + q^2 - 2\left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right)\vec{k}\cdot\vec{q} \,, \end{aligned} $

(40) and replaced in the exponential argument as
$ \begin{aligned}[b] {\lambda_A}_i p_{\lambda_A}^2 + {\lambda_B}_j p_{\lambda_B}^2 + C_l p_C^2 =\;& k^2 \left[ {\lambda_A}_i(1-x)^2 + {\lambda_B}_j \left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right)^2 + C_l \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right)^2\right] + q^2 \left[ {\lambda_A}_i + {\lambda_B}_j + C_l \right] \\ \;&- 2\vec{k}\cdot\vec{q} \left[ {\lambda_A}_i (1-x) + {\lambda_B}_j \left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right) + C_l \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right) \right] \,. \end{aligned} $

(41) Now, to eliminate the
$ \vec{k}\cdot\vec{q} $ term, we fix x to the following value:$ x = \frac{{\lambda_A}_i + {\lambda_B}_j\dfrac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} + C_l\dfrac{\omega_\mu}{\omega_{\eta\mu}}}{{\lambda_A}_i + {\lambda_B}_j + C_l}. $

(42) To simplify the notation further, we define the parameters
$ A = {\lambda_A}_i + {\lambda_B}_j\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} + C_l\frac{\omega_\mu}{\omega_{\eta\mu}} \,, $

(43) $ 2B = {\lambda_A}_i + {\lambda_B}_j + C_l \,, $

(44) $ 2D = {\lambda_A}_i(1-x)^2 + {\lambda_B}_j \left(\frac{\omega_{\mu}}{\omega_{\alpha\beta\mu}} - x\right)^2 + C_l \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x \right)^2 \,, $

(45) where
$ x = \dfrac{A}{2B} $ . Therefore, the linear momentum contribution can be now expressed as$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) =\;& \sum\limits_{ijkl'} d_i^{\lambda_A} d_j^{\lambda_B} (d_k^\rho)^2 d_{l'}^C \int {\rm d}^3q {\rm d}^3k {\rm d}^3p_{\rho} \frac{\delta(k-k_0)}{k^{l+1}} \exp\left( - Bq^2 - Dk^2 -\frac{\rho_k}{2} p_\rho^2 \right) \\ \;&\times \left\{ \left[ \left[{\cal{Y}}_{L_{\lambda_B}}(\vec{p}_{\lambda_B}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_B} {\cal{Y}}_{L_C}(\vec{p}_C) \right]_{L_{BC}} {\cal{Y}}_l(\vec{k}) \right\}_L^* \left\{ \left[{\cal{Y}}_{L_{\lambda_A}}(\vec{p}_{\lambda_A}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_A} {\cal{Y}}_1 \left( \vec{p}\, \right) \right\}_L \,, \end{aligned}$

(46) but the second line of Eq. (46) is still not expressed in terms of the integration variables. Hence, the properties of spherical harmonics and couplings between angular momenta must be used [49] such that
$ \begin{aligned}[b] \left\{ \left[{\cal{Y}}_{L_{\lambda_A}}(\vec{p}_{\lambda_A}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_A} {\cal{Y}}_1 \left( \vec{p} \right) \right\}_L =\;& \sum\limits_{l_1,l_2,l_3,l_4,l_5} B^{l_4}_{l_1,l_2} B^{l_5}_{L_{\lambda_A} - l_1,1 - l_2} C^{L_{\lambda_A}}_{l_1} C^{1}_{l_2} \Pi_{L_A,L_{\lambda_A},l_3,l_4,l_5,1} (1-x)^{l_1} x^{l_2} (-1)^{L + L_A + L_{\lambda_A} - l_1 + 1} k^{l_1+l_2-l_4} q^{L_{\lambda_A} - l_1 - l_2 - l_5 + 1} \\ \;&\times \left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _A}}}}&{{L_\rho }}&{{L_A}}\\ L&1&{{l_3}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_1}}&{{L_{{\lambda _A}}} - {l_1}}&{{L_{{\lambda _A}}}}\\ {{l_2}}&{1 - {l_2}}&1\\ {{l_4}}&{{l_5}}&{{l_3}} \end{array}} \right\} \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[ {\cal{Y}}_{l_4}(\vec{k}) {\cal{Y}}_{l_5}(\vec{q}\,) \right]_{l_3} \right\}_L, \end{aligned} $

(47) where we have defined the following coefficients:
$ {B_{a,b}^c = {{( - 1)}^c}\sqrt {\frac{{(2a + 1)(2b + 1)}}{{4\pi }}} \left( {\begin{array}{*{20}{c}} a&b&c\\ 0&0&0 \end{array}} \right){\mkern 1mu} ,}{\quad \quad }{C_b^a = \sqrt {\frac{{4\pi (2a + 1)!}}{{(2b + 1)!(2(a - b) + 1)!}}} {\mkern 1mu} .} $

(48) The remaining term becomes
$ \begin{aligned}[b] & \left\{ \left[ \left[{\cal{Y}}_{L_{\lambda_B}}(\vec{p}_{\lambda_B}) {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho})\right]_{L_B} {\cal{Y}}_{L_C}(\vec{p}_C) \right]_{L_{BC}} {\cal{Y}}_l(\vec{k}) \right\}_L \\ =\;& \sum\limits_{l_6,l_7,l_8,l_9,l_{10},l_{11},l_{12}} B_{l_6,l_7}^{l_9} B_{L_{\lambda_B} - l_6,L_C - l_7}^{l_{10}} B_{l_9,l}^{l_{12}} C^{L_{\lambda_B}}_{l_6} C^{L_C}_{l_7} \Pi_{L_{BC},L_B,L_C,L_{\lambda_B},l_8,l_8,l_9,l_{10},l_{11},l_{12}}\left(\frac{\omega_\mu}{\omega_{\alpha\beta\mu}} - x\right)^{l_6} \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x\right)^{l_7} \\ & \quad \times (-1)^{L_{BC} + L_B + L_{\lambda_B} + L_\rho + L - l_6 - l_7 + l_{10} + l_{12}} k^{l + l_6 + l_7 - l_{12}} q^{L_{\lambda_B} + L_C - l_6 - l_7 - l_{10}} \left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _B}}}}&{{L_\rho }}&{{L_B}}\\ {{L_{BC}}}&{{L_C}}&{{l_8}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{L_\rho }}&{{l_8}}&{{L_{BC}}}\\ l&L&{{l_{11}}} \end{array}} \right\}\\ & \quad \times \left\{ {\begin{array}{*{20}{c}} {{l_{10}}}&{{l_9}}&{{l_8}}\\ l&{{l_{11}}}&{{l_{12}}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_6}}&{{L_{{\lambda _B}}} - {l_6}}&{{L_{{\lambda _B}}}}\\ {{l_7}}&{{L_C} - {l_7}}&{{L_C}}\\ {{l_9}}&{{l_{10}}}&{{l_8}} \end{array}} \right\} \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[{\cal{Y}}_{l_{12}}(\vec{k}) {\cal{Y}}_{l_{10}}(\vec{q}\,) \right]_{l_{11}} \right\}_L \,. \end{aligned}$

(49) We arrive then at the expression of the linear momentum contribution
$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) =\;& \sum\limits_{ijkl'} d_i^{\lambda_A} d_j^{\lambda_B} (d_k^\rho)^2 d_{l'}^C \int {\rm d}^3q {\rm d}^3k {\rm d}^3p_{\rho} \frac{\delta(k-k_0)}{k^{l+1}} \exp\left( - Bq^2 - Dk^2 -\frac{\rho_k}{2} p_\rho^2 \right) \\ \;&\times \sum\limits_{l_1,l_2,...,l_{11},l_{12}} B^{l_4}_{l_1,l_2} B^{l_5}_{L_{\lambda_A} - l_1,1 - l_2} B_{l_6,l_7}^{l_9} B_{L_{\lambda_B} - l_6,L_C - l_7}^{l_{10}} B_{l_9,l}^{l_{12}} C^{L_{\lambda_A}}_{l_1} C^{1}_{l_2} C^{L_{\lambda_B}}_{l_6} C^{L_C}_{l_7} \\ \;&\times \Pi_{L_{BC},L_A,L_B,L_C,L_{\lambda_A},L_{\lambda_B},l_3,l_4,l_5,l_8,l_8,l_9,l_{10},l_{11},l_{12},1} \, (1-x)^{l_1} x^{l_2} \left(\frac{\omega_\mu}{\omega_{\alpha\beta\mu}} - x\right)^{l_6} \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x\right)^{l_7} \\ \;&\times (-1)^{L_{BC} + L_A + L_B + L_{\lambda_A} + L_{\lambda_B} + L_\rho - l_1 - l_6 - l_7 + l_{10} + l_{12} + 1} k^{l+l_1+l_2-l_4 + l_6 + l_7 - l_{12}} q^{L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_5 - l_6 - l_7 - l_{10} + 1} \\ \;&\times \left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _A}}}}&{{L_\rho }}&{{L_A}}\\ L&1&{{l_3}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _B}}}}&{{L_\rho }}&{{L_B}}\\ {{L_{BC}}}&{{L_C}}&{{l_8}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{L_\rho }}&{{l_8}}&{{L_{BC}}}\\ l&L&{{l_{11}}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_{10}}}&{{l_9}}&{{l_8}}\\ l&{{l_{11}}}&{{l_{12}}} \end{array}} \right\} \end{aligned} $

$ \begin{aligned}[b] \times \left\{ {\begin{array}{*{20}{c}} {{l_1}}&{{L_{{\lambda _A}}} - {l_1}}&{{L_{{\lambda _A}}}}\\ {{l_2}}&{1 - {l_2}}&1\\ {{l_4}}&{{l_5}}&{{l_3}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_6}}&{{L_{{\lambda _B}}} - {l_6}}&{{L_{{\lambda _B}}}}\\ {{l_7}}&{{L_C} - {l_7}}&{{L_C}}\\ {{l_9}}&{{l_{10}}}&{{l_8}} \end{array}} \right\} \times \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[{\cal{Y}}_{l_{12}}(\vec{k}) {\cal{Y}}_{l_{10}}(\vec{q}\,) \right]_{l_{11}} \right\}_L^* \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[ {\cal{Y}}_{l_4}(\vec{k}) {\cal{Y}}_{l_5}(\vec{q}\,) \right]_{l_3} \right\}_L \,, \end{aligned} $

(50) in which the angular integrals can be solved using the orthogonality of spherical harmonics as follows [49]:
$ \begin{aligned}[b] & \int {\rm d}^3q\, {\rm d}^3k\, {\rm d}^3p_{\rho} \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[{\cal{Y}}_{l_{12}}(\vec{k}) {\cal{Y}}_{l_{10}}(\vec{q}\,) \right]_{l_{11}} \right\}_L^* \left\{ {\cal{Y}}_{L_{\rho}}(\vec{p}_{\rho}) \left[ {\cal{Y}}_{l_4}(\vec{k}) {\cal{Y}}_{l_5}(\vec{q}\,) \right]_{l_3} \right\}_L \\ =\;& \delta_{L_\rho,L_\rho} \delta_{l_{12},l_4} \delta_{l_{10},l_5} \delta_{l_{11},l_3} \delta_{L,L} \int {\rm d}q\, {\rm d}k\, {\rm d}p_{\rho}\, q^{2 + l_5 + l_{10}} k^{2 + l_4 + l_{12}} p_{\rho}^{2 + 2L_\rho}. \end{aligned} $

(51) Additionally, the radial integrals can be simplified using the Gamma function when the exponential term is considered. The integral over q becomes
$ \begin{aligned}[b] & \int_0^\infty {\rm d}q\, {\rm e}^{-B q^2} q^{L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_5 - l_6 - l_7 + l_5 + 3} \\ =\;& \frac{1}{2} B^{-\frac{1}{2}(L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_6 - l_7 + 4)} \\ \ \ \ & \times \Gamma\left( \frac{1}{2}(L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_6 - l_7 + 4)\right) \,. \end{aligned}$

(52) The same can be performed for the integral over
$ p_\rho $ :$ \int {\rm d}p_{\rho}\, {\rm e}^{-\frac{\rho_k}{2} p_\rho^2} p_{\rho}^{2 + 2L_\rho} = \frac{1}{2} \left( \frac{\rho_k}{2} \right)^{-\frac{1}{2}(2L_\rho + 3)} \Gamma\left( \frac{1}{2}(2L_\rho + 3)\right) , $

(53) and the integral over k can be simplified using the delta function
$ \delta(k-k_0) $ .Therefore, the lineal momentum contribution has the final expression
$ \begin{aligned}[b] {\cal{E}}(A \rightarrow BC) =\;& \sum\limits_{ijkl'} d_i^{\lambda_A} d_j^{\lambda_B} (d_k^\rho)^2 d_{l'}^C \exp\left( - Dk_0^2 \right) \sum\limits_{l_1,l_2,...,l_8,l_9} B^{l_4}_{l_1,l_2} B^{l_5}_{L_{\lambda_A} - l_1,1 - l_2} B_{l_6,l_7}^{l_9} B_{L_{\lambda_B} - l_6,L_C - l_7}^{l_5} B_{l_9,l}^{l_4} C^{L_{\lambda_A}}_{l_1} C^{1}_{l_2} C^{L_{\lambda_B}}_{l_6} C^{L_C}_{l_7} \\ \;&\times \Pi_{L_{BC},L_A,L_B,L_C,L_{\lambda_A},L_{\lambda_B},l_3,l_3,l_4,l_4,l_5,l_5,l_8,l_8,l_9,1} (1-x)^{l_1} x^{l_2} \left(\frac{\omega_\mu}{\omega_{\alpha\beta\mu}} - x\right)^{l_6} \left(\frac{\omega_\mu}{\omega_{\eta\mu}} - x\right)^{l_7} \\ \;&\times (-1)^{L_{BC} + L_A + L_B + L_{\lambda_A} + L_{\lambda_B} + L_\rho - l_1 - l_6 - l_7 + l_5 + l_4 + 1} (2)^{L_\rho - \frac{1}{2}} k_0^{l_1+l_2 + l_6 + l_7 + 1} B^{-\frac{1}{2}(L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_6 - l_7 + 4)} \rho_k^{-\frac{1}{2}(2L_\rho + 3)} \\ \;&\times \Gamma\left( \frac{1}{2}(L_C + L_{\lambda_A} + L_{\lambda_B} - l_1 - l_2 - l_6 - l_7 + 4)\right) \Gamma\left( \frac{1}{2}(2L_\rho + 3)\right) \left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _A}}}}&{{L_\rho }}&{{L_A}}\\ L&1&{{l_3}} \end{array}} \right\}\\ \;&\times \left\{ {\begin{array}{*{20}{c}} {{L_{{\lambda _B}}}}&{{L_\rho }}&{{L_B}}\\ {{L_{BC}}}&{{L_C}}&{{l_8}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{L_\rho }}&{{l_8}}&{{L_{BC}}}\\ l&L&{{l_3}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_5}}&{{l_9}}&{{l_8}}\\ l&{{l_3}}&{{l_4}} \end{array}} \right\} \left\{ {\begin{array}{*{20}{c}} {{l_1}}&{{L_{{\lambda _A}}} - {l_1}}&{{L_{{\lambda _A}}}}\\ {{l_2}}&{1 - {l_2}}&1\\ {{l_4}}&{{l_5}}&{{l_3}} \end{array}} \right\}\left\{ {\begin{array}{*{20}{c}} {{l_6}}&{{L_{{\lambda _B}}} - {l_6}}&{{L_{{\lambda _B}}}}\\ {{l_7}}&{{L_C} - {l_7}}&{{L_C}}\\ {{l_9}}&{{l_5}}&{{l_8}} \end{array}} \right\} \,, \end{aligned} $

(54) where the limits of the sums can be obtained using triangular conditions of the Wigner symbols.
-
The color matrix element can be expressed as
$ {\cal{I}}_{\rm Color} = \langle C_B C_C | C_A \rangle = \langle C(\epsilon\sigma\zeta) C(\delta\tau) | C(\alpha\beta\eta) C(\mu\nu) \rangle \,, $

(55) where the color function of the pair created is added. To calculate this contribution, we must know the color function of the baryons and mesons:
$ \begin{aligned}[b] C(\alpha\beta\eta) = \;& \frac{1}{\sqrt{6}} \sum\limits_{\alpha\beta\eta} \varepsilon_{\alpha\beta\eta} = \frac{1}{\sqrt{6}} (r_\alpha g_\beta b_\eta - r_\alpha b_\beta g_\eta \\ & + g_\alpha b_\beta r_\eta - g_\alpha r_\beta b_\eta + b_\alpha r_\beta g_\eta - b_\alpha g_\beta r_\eta) \end{aligned} \,, $

(56) $ C(\delta\tau) = \frac{1}{\sqrt{3}} \sum\limits_{\delta\tau} \delta_{\delta\tau} = \frac{1}{\sqrt{3}}(r_\delta \overline{r}_\tau + g_\delta \overline{g}_\tau + b_\delta \overline{b}_\tau) \,. $

(57) Because mesons are composed of a quark and antiquark, a Kronecker delta is sufficient to describe the color function. In contrast, the baryon must have an antisymmetric color wave function, making the Levi-Civita symbol sufficient to describe the color of the system.
The color wave functions are replaced in Eq. (55), and considering the particle equivalences of Eq. (12), the color contribution becomes
$ \begin{aligned}[b] {\cal{I}}_{\rm Color} =\;& \frac{1}{18} \sum\limits_{\alpha\beta\eta} \sum\limits_{\mu\nu} \sum\limits_{\epsilon\sigma\zeta} \sum\limits_{\delta\tau} \varepsilon_{\alpha\beta\eta} \delta_{\mu\nu} \varepsilon_{\epsilon\sigma\zeta} \delta_{\delta\tau} \\ =\;& \frac{1}{18} \sum\limits_{\alpha\beta\eta\mu} \sum\limits_{\epsilon\sigma\zeta\delta} \varepsilon_{\alpha\beta\eta} \varepsilon_{\epsilon\sigma\zeta} \delta_{\delta\mu} \delta_{\epsilon\mu} \delta_{\zeta\alpha} \delta_{\sigma\beta} \delta_{\delta\eta} \\ =\;& \frac{1}{18} \sum\limits_{\alpha\beta\eta} \sum\limits_{\delta} \varepsilon_{\alpha\beta\eta} \varepsilon_{\delta\beta\alpha} \delta_{\delta\eta} \\ =\;& \frac{1}{18} \sum\limits_{\alpha\beta\eta} \varepsilon_{\alpha\beta\eta} \varepsilon_{\eta\beta\alpha} \,. \end{aligned} $

(58) Now, the product of Levi-Civita tensors can be simplified as
$ \sum\limits_{\alpha\beta\eta} \varepsilon_{\alpha\beta\eta} \varepsilon_{\eta\beta\alpha} = -6 \,, $

(59) arriving at
$ {\cal{I}}_{\rm Color} = -\frac{1}{3} \,. $

(60) This term cancels with the
$ 3 $ placed by hand in the transition operator, Eq. (1). -
The flavor matrix element may be expressed as
$ {\cal{I}}_{\rm Sabor} = \langle [(t_\mu t_\beta t_\alpha)I_B(t_\nu t_\eta)I_C]I_A | [(t_\alpha t_\beta t_\eta)I_A (t_\mu t_\nu) 0]I_A \rangle \,. $

(61) To simplify this expression, we define the flavor of the non-interacting quarks (α and β) inside baryons similar to that for the spin:
$ t_\rho = t_\alpha \otimes t_\beta. $

(62) Thus, the final expression to be calculated is
$ \begin{split} {\cal{I}}_{\rm Sabor} =\;& (-1)^{t_\alpha + t_\beta + t_\mu - I_B} (-1)^{t_\nu + t_\eta - I_C} \\ &\times \langle [(t_\rho t_\mu)I_B(t_\eta t_\nu)I_C]I_A | [(t_\rho t_\eta)I_A (t_\mu t_\nu) 0]I_A \rangle \,. \end{split} $

(63) This can be re-written using a
$ 9J $ -symbol as$ {\cal{I}}_{\rm Sabor} = (-1)^{t_\alpha + t_\beta + t_\mu - I_B} (-1)^{t_\nu + t_\eta - I_C} \Pi_{I_B,I_C,I_A,0}\left\{ {\begin{array}{*{20}{c}} {{t_\rho }}&{{t_\mu }}&{{I_B}}\\ {{t_\eta }}&{{t_\nu }}&{{I_C}}\\ {{I_A}}&0&{{I_A}} \end{array}} \right\} \,. $

(64) Because the
$ 9J $ -symbol has a zero in one of its components, it can be simplified into a$ 6J $ -symbol:$ {\cal{I}}_{\rm Sabor} = (-1)^{t_\alpha + t_\beta + t_\mu + t_\eta + t_\rho + I_A - I_B} \frac{\Pi_{I_B,I_C}}{\Pi_{t_\mu}} \left\{ {\begin{array}{*{20}{c}} {{t_\eta }}&{{I_C}}&{{t_\mu }}\\ {{I_B}}&{{t_{{\rho _A}}}}&{{I_A}} \end{array}} \right\}, $

(65) where the equivalence of
$ t_\mu = t_\nu $ is used. -
After the detailed derivation of the analytical expression for the decay width and transition matrix of a baryon decaying strongly into a meson plus another baryon, we now provide an example of calculation to check its correctness. Moreover, one of our long-term objectives is to provide a unified picture of mesons and baryons decaying strongly, from our previous analysis in the meson sector [21].
The most convenient example for our test is the
$ \Delta(1232) $ baryon decaying strongly into a pion ($ \pi(140) $ ) plus a nucleon ($ N(940) $ ). This is because (i) all hadrons involved in the reaction are well established in the Particle Listings of the PDG [1]; (ii) the two hadrons in the final state are stable, avoiding additional complications in the computation related by considering decay widths of the products; (iii) all constituent quarks inside hadrons are either u- or d-quarks and, because isospin symmetry is well fulfilled in QCD, we can assume all of them as equivalent quarks; (iv) the branching fraction of the$ \Delta(1232)\to \pi N $ strong decay channel is 99.4%, which constitutes almost the total decay width; (v) the total decay width is relatively well measured experimentally, with a value between$ 114\;\text{MeV} $ and$120\;\text{MeV}$ [1].2 As mentioned earlier, the Δ-baryon is composed of up (u) and down (d) quarks in different combinations, which results in
$ 4 $ different species with different charges and decay channels. This species and their properties are given in the following table:Baryon Quarks Charge (in units of e) Decay Channels $ \Delta^{++} $ 

uuu +2 $ p^+ $ +

$ \pi^+ $ 

$ \Delta^{+} $ 

uud +1 $ n^0 $ +

$ \pi^+ $ or

$ p^+ $ +

$ \pi^0 $ 

$ \Delta^{0} $ 

udd 0 $ n^0 $ +

$ \pi^0 $ or

$ p^+ $ +

$ \pi^- $ 

$ \Delta^{-} $ 

ddd −1 $ n^0 $ +

$ \pi^- $ 

To calculate the decay width, we must fix the properties of the initial and final hadrons. For simplicity and without loss of generality, the studied decaying baryon is
$ \Delta(1232)^- $ . Therefore, the properties of all the hadrons involved in the reaction are listed as follows (a constituent quark model description of hadrons is assumed):Hadron $ {L} $ 

$ {S} $ 

$ {J} $ 

Mass/MeV Radius/fm Δ $ 0 $ 

$ 3/2 $ 

$ 3/2 $ 

$ 1232 $ 

$ 1.03 $ 

n $ 0 $ 

$ 1/2 $ 

$ 1/2 $ 

$ 940 $ 

$ 0.84 $ 

$ \pi^{+/-} $ 

$ 0 $ 

$ 0 $ 

$ 0 $ 

$ 140 $ 

$ 0.5 $ 

Using the experimental masses, the transferred momentum of the reaction can be calculated:
$ k_0 = \frac{\sqrt{\left(m_A^2 - (m_B - m_C)^2\right)\left(m_A^2 - (m_B + m_C)^2\right)}}{2m_A} = 226\,\text{MeV} \,. $

(66) Having fixed the target reaction to be studied, let us now determine some relevant couplings required to provide a final numerical result. For example, the coupling of angular momenta in the final state can be deduced as follows:
$ \ {{J_{BC}} = {J_B} \otimes {J_C} = \frac{1}{2}}\quad {{\rm{and}}}\quad{{L_{BC}} = {L_B} \otimes {L_C} = 0{\mkern 1mu} .} $

(67) For the other values, slightly more work is necessary. For instance, the baryons have two internal momenta that result from the
$ (\rho\lambda) $ -Jacobi coordinates; they should fulfill$ \begin{array}{l} L_{\lambda_A} \otimes L_\rho = 0 \,, \\ L_{\lambda_B} \otimes L_\rho = 0 \,, \end{array} $

(68) which dictate that
$ L_{\lambda_A} = L_{\lambda_B} = L_\rho \,, $

(69) and we assume that all of them are zero. Additionally, the final hadrons have a relative angular momentum, l, which must be considered to assure the conservation of angular momentum,
$ \vec{J}_A = \vec{J}_{BC} + \vec{l} \quad \Rightarrow \quad \frac{3}{2} = \left|\frac{1}{2} \otimes l\right|, $

(70) having two possible values:
$ l = 1 \text{ or } l = 2 \,. $

(71) During the calculation, the Wigner symbols eliminate any of the values that do not comply with the triangular conditions.
Regarding spin coupling, the quarks are fermions with a spin of
$ 1/2 $ , and$ s_\rho $ must be maintained as it is the total spin of the two spectator quarks. Therefore, the next decomposition is performed:$\begin{aligned}[b] \;& S_A = \frac{3}{2} = \left( \frac{1}{2} \otimes \frac{1}{2}\right)_1 \otimes \frac{1}{2} = \left( s_\alpha \otimes s_\beta \right)_{s_\rho} \otimes s_\eta \,, \\ \;&S_B = \frac{1}{2} = \left( \frac{1}{2} \otimes \frac{1}{2}\right)_1 \otimes \frac{1}{2} = \left( s_\alpha \otimes s_\beta \right)_{s_\rho} \otimes s_\mu \,, \\ \;&S_C = 0 = \frac{1}{2} \otimes \frac{1}{2} = s_\eta \otimes s_\nu \,. \end{aligned} $

(72) Note that the spin of the spectator quarks must be equal to
$ 1 $ to correctly provide the Δ quantum numbers. Thus, this requires the same value of$ s_\rho $ in the nucleon. Similar reasoning is made for the isospin couplings:$\begin{aligned}[b] & I_A = \frac{3}{2} = \left( \frac{1}{2} \otimes \frac{1}{2}\right)_{1} \otimes \frac{1}{2} = \left( t_\alpha \otimes t_\beta \right)_{t_\rho} \otimes t_\eta \,, \\ & I_B = \frac{1}{2} = \left( \frac{1}{2} \otimes \frac{1}{2}\right)_{1} \otimes \frac{1}{2} = \left( t_\alpha \otimes t_\beta \right)_{t_\rho} \otimes t_\mu \,, \\ & I_C = 1 = \frac{1}{2} \otimes \frac{1}{2} = t_\eta \otimes t_\nu \,. \end{aligned}$

(73) To estimate the coefficients that appear in the Gaussian expansion of the hadron’s wave functions, we must perform some analysis. The Gaussian form for the meson can be assumed to be
$ f(\vec{p}_C) = d^C {\rm e}^{-\frac{C}{2}p^2_C} \,, $

(74) where only one term of the sum is used to simplify the calculation. The form of the Gaussian function provides the next relation between the variance and the coefficient:
$ \sigma^2 = \frac{1}{C} \,. $

(75) Thus, the hadron’s radius can be approximated as
$ \langle r^2 \rangle \approx \frac{(\hbar c)^2}{\sigma^2}, $

(76) where
$ \hbar c = 0.197327\,\text{GeV fm} $ is included to obtain the correct units. This is the relationship we will use between the hadron’s coefficient and its size.The Gaussian expansion for a baryon is different because it has two components. Following Ref. [50] the next function is used:
$ f(\vec{p}_\lambda,\vec{p}_\rho) = \left[ \frac{2b^2}{\pi} \right]^{{3}/{4}} {\rm e}^{-b^2\vec{p\,}_\rho^2} \left[ \frac{2\alpha b^2}{\pi} \right]^{{3}/{4}} {\rm e}^{-\alpha b^2\vec{p\,}_\lambda^2} \,, $

(77) where α depends on quark masses as
$ \alpha = \frac{m_1m_2(m_1 + m_2 + m_3)}{m_3(m_1 + m_2)^2} \,, $

(78) which is equal to
$ 3/4 $ in our case. Equation (77) shows us two important aspects: (i) the computation of the baryon radius in terms of its Gaussian's standard deviation and (ii) the relation$ d^C = \left[\frac{2C}{\pi}\right]^{3/4} \,. $

(79) Now, we can compute the coefficients necessary for the transition matrix (see Eqs. (42)−(45)),
$\qquad A = \frac{9\langle r_A \rangle^2 + 3\langle r_B \rangle^2 + 2\langle r_C \rangle^2}{4(\hbar c)^2} \,, $

(80) $ \qquad B = \frac{9\langle r_A \rangle^2 + 9\langle r_B \rangle^2 + 4\langle r_C \rangle^2}{8(\hbar c)^2} \,, $

(81) $ x = \frac{9\langle r_A \rangle^2 + 3\langle r_B \rangle^2 + 2\langle r_C \rangle^2}{9\langle r_A \rangle^2 + 9\langle r_B \rangle^2 + 4\langle r_C \rangle^2} \,, $

(82) $ \begin{aligned}[b] D =\;& \frac{1}{2}\left[ \frac{9\langle r_A \rangle^2}{4(\hbar c)^2}\left(1-x \right)^2 + \frac{9\langle r_B \rangle^2}{4(\hbar c)^2} \left(\frac{1}{3} - x\right)^2 \right.\\ & \left.+ \frac{\langle r_C \rangle^2}{(\hbar c)^2}\left(\frac{1}{2} - x\right)^2 \right] \,, \end{aligned} $

(83) and the product of the amplitudes,
$ d_i^{\lambda_A} d_i^{\lambda_B}(d_k^\rho)^2 d_l^C = \rho_k^{{3}/{2}} \left[\left(\frac{2}{\pi}\right)^{5} \left(\frac{81\langle r_A \rangle^2 \langle r_B \rangle^2 \langle r_C \rangle^2}{16(\hbar c)^6} \right) \right]^{{3}/{4}} \,. $

(84) Note that
$\rho_k^{{3}/{2}}$ cancels with the$\rho_k^{-{3}/{2}}$ that appears in the spin-space component, Eq. (54).The final constant that must be known is the only-free paramter of the
$ {}^3P_0 $ decay model,$ \gamma' $ , that characterizes the strength of the quark-antiquark pair creation from the vacuum. Following Ref. [21], the next relation can be used to calculate this constant:$ \gamma' = \sqrt{2^5 \pi} \,\cdot \frac{\gamma_0}{\log\left(\frac{\mu}{\mu_\gamma}\right)}, $

(85) where
$ \gamma_0 = 0.81\pm0.02 $ and$ \mu_\gamma = 49.84\pm2.58\,\text{MeV} $ are constants fitted to the total strong decay widths of mesons, and μ represents the quark sector to which the decaying hadron belongs. For the example at hand, the Δ-baryon belongs to the light quark sector. Therefore, following Ref. [21], we obtain$ \mu = m_q/2 = 313\,\text{MeV}/2 = 156.5\,\text{MeV} $ , where$ m_q $ is the value of the constituent light quark mass in our model, and$ \gamma' = \sqrt{16\pi} \approx 7.09 \,. $

(86) All together, they provide the following value for the decay width:
$ \Gamma({\Delta(1232)^- \to n(940)+\pi(140)^-}) = 113.32~ \text{MeV} \,, $

(87) which is just at, or below, the minimum given by the PDG [1], viz.
$ \Gamma_{\Delta(1232)} = (114-120)\;\text{MeV} $ . Therefore,$\Gamma_{\Delta(1232)\to N\pi} = (113-119)\;\text{MeV}$ . This result is remarkable because, in some sense, it is a free-parameter prediction of the$ {}^3P_0 $ decay model using only the experimental values of the hadron’s radii reported in Ref. [1] and the scale-dependent strength determined in Ref. [21].To contrast this result, the
$ \gamma' $ value often used in the literature is the one obtained in the fitting performed by Blundell [29]. Because this value is fixed for meson decays, it must be divided by$ \sqrt{3} $ to extrapolate it for baryon decays.3 Therefore, the next value of$ \gamma' $ can be used:$ \gamma' = \frac{13.4}{\sqrt{3}}, $

(88) having a decay width of
$ \Gamma({\Delta(1232)^- \to n(940)+\pi(140)^-}) = 134.95~\text{MeV} \,, $

(89) which is slightly higher than the experimental interval but relatively correct [1], confirming that the analytical development of the
$ {}^3P_0 $ model for baryon decays appears to be correct, and the radii of the involved hadrons are well estimated.Note herein that, because this is a strong Δ-baryon decay, the only variation among charge channels lies in the corresponding Clebsch-Gordan coefficient and possible final states. Theoretically, this implies that each charge channel can be calculated independently. However, from an experimental perspective, when one channel is known, the others are of no practical interest. This characteristic is evident in the PDG, where the differences in the
$ \Delta\to \pi N $ strong decay due to the possible charge states are not documented, and a single value is provided. -
This work have shown in detail how to obtain the transition matrix element for a baryon decaying strongly into a meson and another baryon through the well-known
$ ^3P_0 $ quark-antiquark pair creation model.Because one of our long-term objectives is to provide a unified picture of mesons and baryons from our chiral quark model, an important feature is to describe meson and baryon strong decays under the same umbrella. Such a study was conducted on the meson in Ref. [21], and we have sought to extend the same formalism to the baryon sector, focusing on the
$ \Delta(1232)\to \pi N $ strong decay width because all hadrons involved in the reaction are very well established, the two hadrons in the final state are stable and require no further analysis, all quarks are light and equivalent, and the decay width of the process is relatively well measured.We can conclude that the core concept of the
$ {}^3P_0 $ decay model remains robust: the dominant strong decays of mesons – and by extension, baryons – are driven by the creation of a quark-antiquark pair from the vacuum with quantum numbers$ J^{PC} = 0^{++} $ , followed by the recombination of this quark and antiquark into separate final states. While we aim for the$ {}^3P_0 $ model to capture at least the most fundamental aspects of all strong decays of mesons and baryons, we acknowledge that fully describing the finer details is a challenging, if not unattainable, task. This is the essence of what we mean by ''unification.''Utilizing a Gaussian expansion method for the hadron’s radial wave functions, the expression of the invariant matrix element can be simplified into a sum of multiple terms composed basically on some numerical values, wave function coefficients, and Wigner symbols. Those wave function coefficients can be determined from the mean-square radii of involved hadrons, and we have used their experimental measures in such that the only one free parameter is the strength of the quark-antiquark pair creation from the vacuum. This has been obtained from our previous study on strong decay widths in the meson sector [44], and we have obtained a reasonably compatible result with the experiment for the calculated
$\Delta(1232)\to \pi N$ decay width.The past two decades have been a golden age of meson spectroscopy. Worldwide experimental facilities have reported many new exotic charmonium- and bottomonium-like hadrons, which are collectively called XYZ states, whose nature is still unclear. It is expected that the same puzzling scenario will appear in the baryon sector in the near future, with some pentaquark signals already reported by the LHCb@CERN. This work has been developed in setting one of the three steps towards a novel raft of quark model applications to exotic baryons. Within the quark model framework, exotic baryons as meson-baryon molecules should be explained by basically the diagonalization of a matrix that has three blocks: (i) baryon spectrum, (ii) meson-baryon states formed by the residual interactions between the quarks that constitute the involved hadrons, and (iii) the coupling of baryon and meson-baryon sectors through, for instance, the
$ {}^3P_0 $ decay model. This is the context in which our derivation of the$ {}^3P_0 $ decay model for baryons acquires its relevance, with some steps already performed for the meson-baryon block [51], and we are researching the first item in which baryon wavefunctions will be obtained.
Detailed derivation of the 3P0 strong decay model applied to baryons
- Received Date: 2024-05-06
- Available Online: 2025-01-15
Abstract: By using the






 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF




















 DownLoad:
DownLoad: