-
In [1], we examined the effects of dark energy on the gravitational energy for the quintessential charged-Kerr black holes by approximate Lie symmetry approach. It was shown that the gravitational energy of the charged-Kerr black hole spacetime surrounded by dark energy decreased due to the presence of the cosmological constant (
$ {\omega}_{c}=-1 $ ) and quintessence dark energy ($ {\omega}_{q}=-\dfrac{2}{3} $ ) [1]. Whereas, for the case of frustrated network of cosmic strings ($ {\omega}_{n}=-\dfrac{1}{3} $ ) the contribution in energy of the charged-Kerr black hole due to the presence of dark energy term$ E_{n} $ was observed to be positive for different values of dark energy parameter α (as shown in FIG. 7.) while FIG. 8. shows the effect of dark energy with different values of spin a. We observed there, that if the dark energy parameter α is initially small, then the value of$ E_{n} $ will increase primarily and then it showed a gradual decline for the larger values of α [1]. This result needs correction.Therefore in this corrigendum, we present the effects of dark energy on the energy content of the charged-Kerr black hole surrounded by the dark energy for
$ {\omega}_{n}=-\frac{1}{3} $ . It is observed that at this value of the equation of state parameter, the presence of the dark energy first decreases the energy content of the charged-Kerr black hole surrounded by the dark energy and then increases the energy. This is shown by the graphical results. -
As shown in [1], in the case of
$ {\omega}_{n}=-\frac{1}{3} $ , we get the following re-scaling factor of energy for the charged-Kerr spacetime surrounded by dark energy$ M_{C-K-n}=\frac{M}{2{r}}\Bigg[1-\frac{{Q}^2}{2{M}^2}\Bigg]+\frac{3Ma}{4r^{2}}+\frac{M\alpha}{2r}\Bigg[\frac{1}{1-\alpha}-\frac{a}{2r}\Bigg] $

(1) It was observed, for
$ {\omega}_{n}=-\frac{1}{3} $ , the total energy in the charged-Kerr spacetime surrounded by dark energy varies from the energy in the charged-Kerr spacetime by the following expression [1]$ {E}_{n}=\frac{M\alpha}{2r}\Bigg[\frac{1}{1-\alpha}-\frac{a}{2r}\Bigg] $

(2) In order to analyze the significant features of dark energy, we sketch the expression
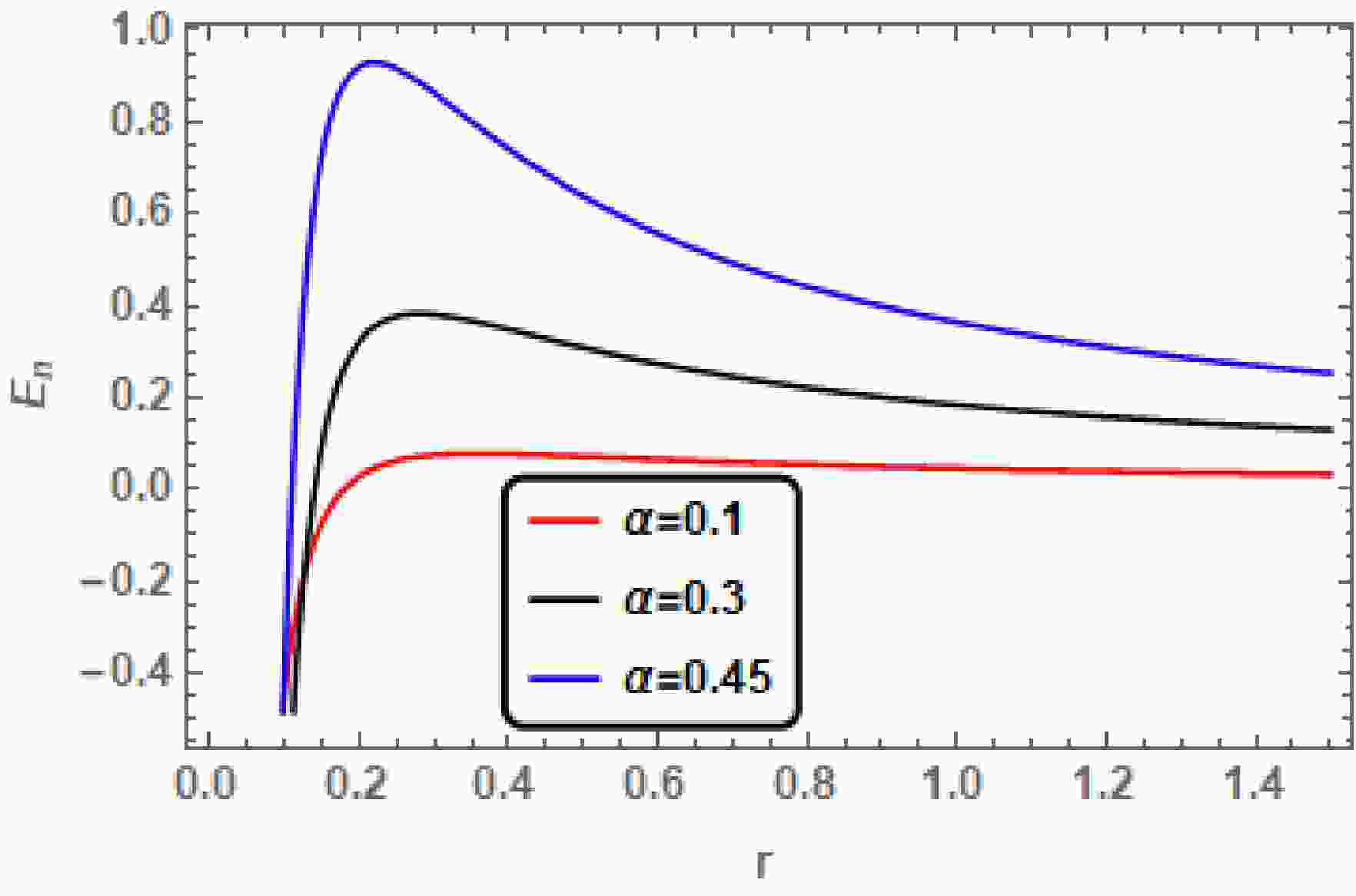
$ (2) $ versus α and radial distance r.From FIG. 1, it is observed that for some values of α and for smaller values of r the value of
$ E_{n} $ is negative which shows that it decreases the energy content of the underlying spacetime, and for some values of α and relatively bigger values of r, the value of$ E_{n} $ is positive and hence increases the energy content of the CK spacetime (as evident from (1)). Hence, we observe that the contribution of dark energy term for$ {\omega}_{n}=-\dfrac{1}{3} $ , first decreases the total energy of the underlying spacetime for smaller values of radial coordinate r and then increases for comparatively larger values of r. FIG. 2 depicts the increasing behavior of$ E_{n} $ for different values of spin parameter a.
Figure 1. (color online) plots showing the behavior of
$ {E}_n $ for the quintessential charged-Kerr black hole for$ {\omega}_{n}=-\dfrac{1}{3} $ with different values of α and a fixed value of$ M=1 $ ,$ a=0.4 $ .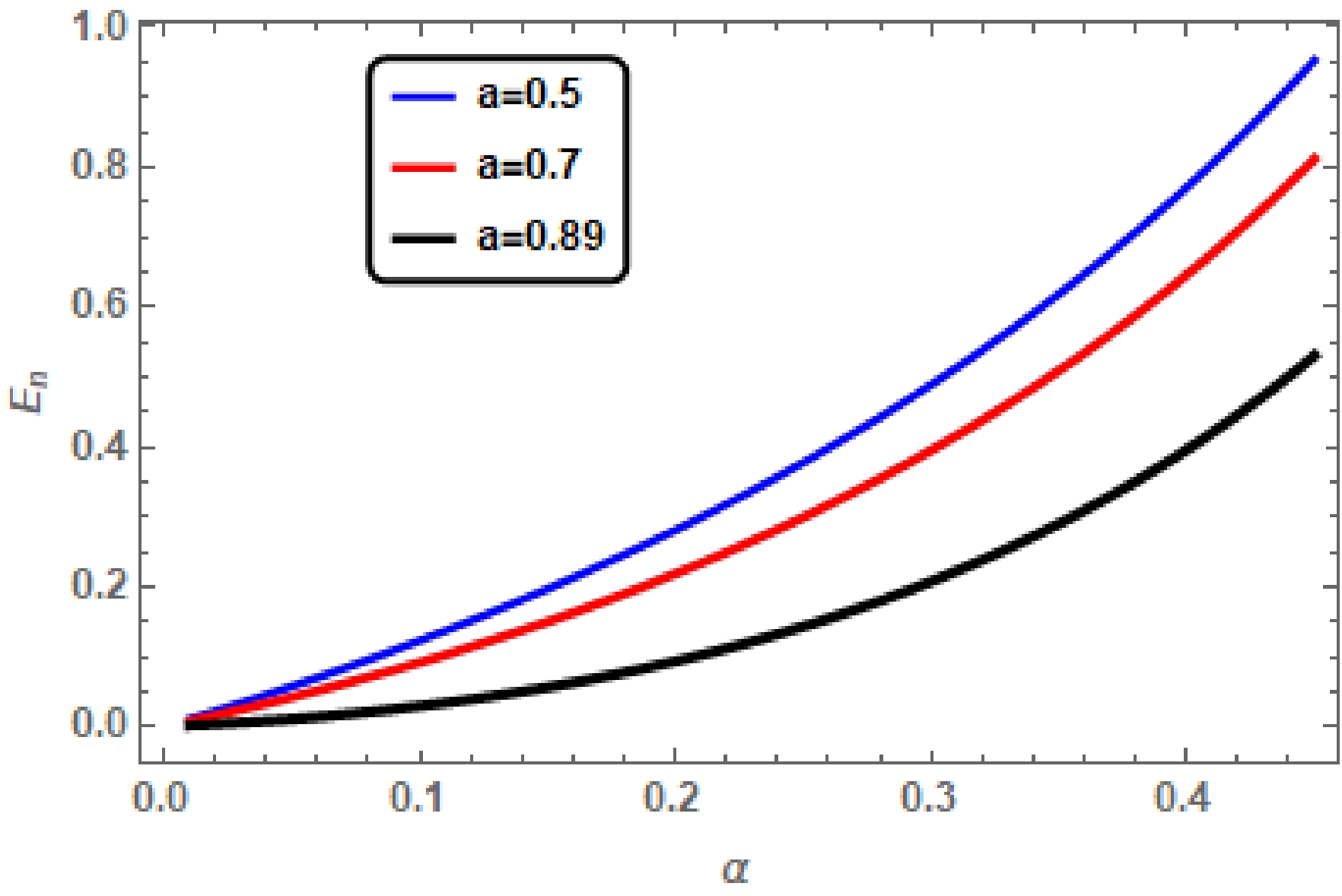
Figure 2. (color online) plots showing the behavior of
$ {E}_n $ for the quintessential charged-Kerr black hole for$ {\omega}_{n}=-\dfrac{1}{3} $ with different values of a,$ 0<\alpha<1/2 $ and a fixed value of$ M=1 $ and$ r=0.4 $ .Therefore, in view of the above discussion it is concluded that the result given in [1] must be stated as
Remark: The effect of cosmological constant and quintessence decreases the energy of the charged-Kerr black hole surrounded by dark energy, whereas the effect of a frustrated network of cosmic strings decreases the energy of the charged-Kerr black hole surrounded by dark energy for some values of α and for smaller values of r and increases the energy for some values of α and relatively bigger values of r as evident from
$ (1) $ .
Erratum: Corrigendum to "Effect of dark energy models on the energy content of charged and rotating black holes" [Chinese Phys. C, 46 (2022) 015101]
- Received Date: 2023-08-18
- Available Online: 2023-12-15
Abstract: In Liaqat and Hussain (2022) it was proved that the effect of dark energy with





 Abstract
Abstract HTML
HTML Reference
Reference Related
Related PDF
PDF














 DownLoad:
DownLoad: