Monopole effects and high-spin levels in neutron-rich ¹³²Te

I. INTRODUCTION

• I. INTRODUCTION
• II. HAMILTONIAN AND MONOPOLE EFFECTS
• III. HIGH-SPIN LEVELS
• IV. CONCLUSION

The analysis of neutron-rich isotopes near the doubly magic nucleus $^{132}$Sn can provide fascinating findings related to nuclear physics and nuclear astrophysics. The abundance peak appears at $A\sim130$, which is formed through the rapid neutron capture process [1, 2]. The properties of doubly magic $^{132}$Sn have been explored and confirmed in both experiments and theories [3−8]. In this nuclei region, tellurium isotopes have attracted experimental and theoretical research interest [5, 9−13]. For example, the g-factor of $^{134}$Te in the 4$^+$ state was measured, which provides direct insight into the single particle structure [9]. The state (17$^+$) of $^{132}$Te was observed to be 6.166 MeV using the reaction $^{9}$Be($^{238}$U, f ) with a beam energy of 6.2 MeV/u at GANIL [14]. A significant energy difference exists in this state between experiments and shell-model calculations, which should conceal unknown information about the nuclear structure.

In theory, the extended pairing plus multipole-multipole force and monopole correction terms model (EPQQM) provides a suitable method to describe both the low-lying states and core excitations [15−18]. For example, the ordering and energies of the low-lying isomers in $^{129}$Cd are predicted and determined by using the implemented phase-imaging ion-cyclotron-resonance method [18, 19]. The 16$^+$ level in $^{128}$Cd is predicted as a spin-trap isomer feeding the known 16$^+$ of $^{128}$In through $\beta^-$ decay [20]. In addition to monopole interactions, it is necessary to consider the cross-shell excitations to study the properties of these neutron-rich nuclei in this region. For example, identifying the isomer state of level 19/2$^+$ at 1942 keV in $^{133}$Ba requires the cross-shell orbits lying above the energy gap $N = 82$. As reported in Ref. [21], the interactions without core excitations cannot provide the B(E1) value for the transition from $J^{\pi} = 19/2^+$ to $J^{\pi} = 19/2^-$.

This model has an advantage for studying monopole effects by employing monopole correction (Mc) terms. For example, in the southwest quadrant ($Z \leq 50, N \leq 82$) of $^{132}$Sn, the level spectra and the energy gap across $N = 82$ can be modified by monopole correction between neutron orbit $h_{11/2}$ and $f_{7/2}$ [15]. In the northeast quadrant ($Z \geq 50, N \geq 82$), five monopole terms are used to describe core excitations and high-spin levels, and the states 2$^-$ and 9$^-$ in $^{136,138}$Te are predicted as a spin-trap structure coupled by the neutron intruder orbit $i_{13/2}$ [22]. Different effects of tensor forces are also discussed together with the monopole-driven shell evolutions [23], as well as other ones in the nucleon-nucleon interaction [24−27]. For the particle-hole nuclei in the northwest region of $^{132}$Sn, a suitable interaction has been found, and the spectra of Sb and Te isotopes are well described as single-orbital couplings and cross-shell excitations [18, 28]. The transition probabilities in these nuclei are also calculated and reproduced well through comparisons with the known data.

In this study, we investigate high-spin levels and monopole effects in particle-hole nuclei near $^{132}$Sn by employing the interaction in Ref. [18]. The shell-model code NUSHELLX@MSU is used for the calculations [29].

II. HAMILTONIAN AND MONOPOLE EFFECTS

• I. INTRODUCTION
• II. HAMILTONIAN AND MONOPOLE EFFECTS
• III. HIGH-SPIN LEVELS
• IV. CONCLUSION

In this work, we use the Hamiltonian in the proton-neutron ($pn$) representation [18]:

Equation (1) includes the single-particle Hamiltonian ($H_{\rm sp}$); the $J=0$ and $J=2$ pairings ($P_{0}^{\dagger}P_{0}$ and $P_{2}^{\dagger}P_{2}$); the quadrupole-quadrupole ($Q^{\dagger}Q$), octupole-octupole ($O^{\dagger}O$), and hexadecapole-hexadecapole ($H^{\dagger}H$) terms; and the monopole corrections ($H_{\rm mc}$). In the $pn$-representation, $P^\dagger_{JM,ii'}$ and $A^\dagger_{JM}(ia,i'c)$ are the pair operators, while $Q^\dagger_{2M,ii'}$, $O^\dagger_{3M,ii'}$, and $H^\dagger_{4M,ii'}$ are the quadrupole, octupole, and hexadecapole operators, respectively, in which i ($i'$) is an index for protons (neutrons). The parameters $g_{J,ii'}$, $\chi_{2,ii'}$, $\chi_{3,ii'}$, $\chi_{4,ii'}$, and $k_{\rm mc}(ia,i'c)$ are the corresponding force strengths, and b is the harmonic-oscillator range parameter.

The model space includes five orbits $(0g_{7/2}, 1d_{5/2}, 1d_{3/2}, 2s_{1/2}, 0h_{11/2})$ for both protons and neutrons. Two extra neutron orbits above the $N=82$ shell, i.e., $(1f_{7/2}$ and $2p_{3/2})$, are added to allow neutron cross-shell excitations. We keep the same parameters of single-particle energies and the two-body force strengths used in Ref. [18]. The monopole interactions were found to be crucial for describing nuclear properties, which are entirely responsible for global saturation properties and single-particle behavior. The neutron-rich nuclei near $^{132}$Sn can be divided into four quadrants by the crossing of $Z = 50$ and $N = 82$. According to nuclei studied previously [15, 22, 30], the monopole corrections are necessary for the hole (particle) nuclei in the southwest (northeast) quadrant of $^{132}$Sn.

In southwest quadrant ($Z \leq 50, N \leq 82$), the ground state inversion in $^{129}$Cd can be well described by the monopole correction between proton orbit $0g_{9/2}$ and neutron orbit $0h_{11/2}$ with a strength of –0.40 MeV [17], and it was verified using the recently implemented phase-imaging ion-cyclotron-resonance method [19]. Recently, the ground-state inversions from $N = 81$ to $N = 79$ were explained for the first time by monopole correction between neutron orbits $h_{11/2}$ and $d_{3/2}$. Furthermore, this monopole correction has been found in different isotonic chains of $N = 79,80,81$, as all being hole nuclei near $^{132}$Sn.

In the northeast quadrant ($Z \geq 50, N \geq 82$), five monopole terms are used to describe core excitations and high-spin levels [22]. For particle-pole nuclei in the northwest quadrant of $^{132}$Sn ($Z \geq 50, N \leq 82$), the protons and neutrons occupy the same major shell. The properties of particle-hole nuclei can be well described without additional monopole correlations [28]. Such a situation is confirmed by the electromagnetic transitions of Sb and Te isotopes in the northwest quadrant of $^{132}$Sn [28, 31], which is a strict test for shell model calculations. However, in level 17$^+$ of $^{132}$Te, the large difference between experiment and theory motivates us to investigate monopole interactions in the particle-hole nuclei region near $^{132}$Sn.

III. HIGH-SPIN LEVELS

• I. INTRODUCTION
• II. HAMILTONIAN AND MONOPOLE EFFECTS
• III. HIGH-SPIN LEVELS
• IV. CONCLUSION

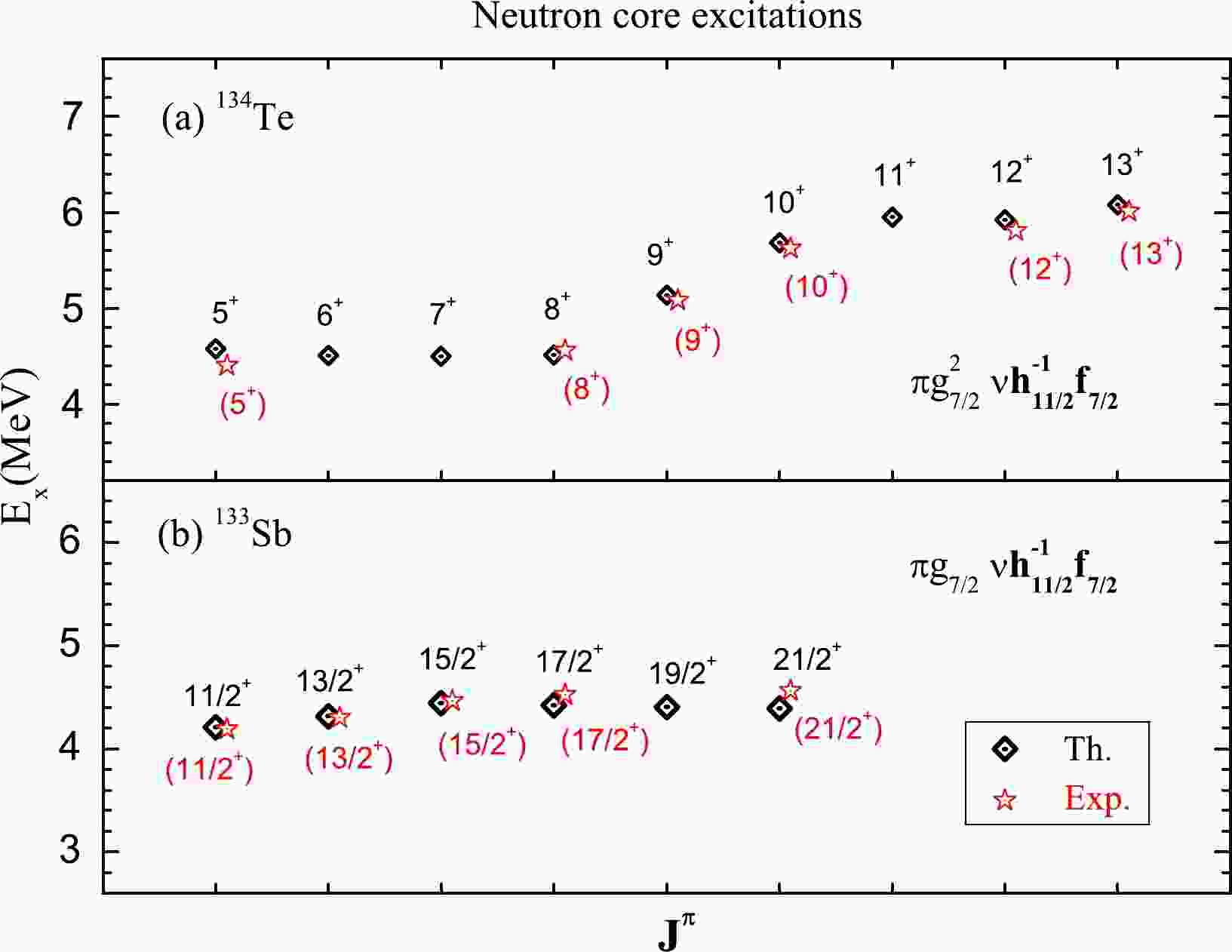
In this part, high-spin levels are investigated with the monopole effects and quadruple-quadruple force in $^{132-134}$Te, $^{131-133}$Sb, and $^{130}$Sn. The monopole correction alone cannot solve the puzzle of level 17⁺; it should be combined with quadrupole correction. As shown in Table 1, there are four different types of level 17$^+$ under 10 MeV according to the EPQQM model, i.e., Nos. 1 to 4. The possibility of neutron core excitation (config.1 in Table 1) at 8.189 MeV can be excluded to explain the high-spin level 17$^+$ of $^{132}$Te [28]. The given experimental data of $^{134}$Te and $^{133}$Sb have been reproduced very well as core excitations with a common neutron configuration of $\nu h^{-1}_{11/2} f_{7/2}$ (Fig. 1). If we modify the monopole term of Mc($\nu h^{-1}_{11/2}, \nu f_{7/2}$) to explain the level 17$^+$ of $^{132}$Te, the 17 states of neutron core excitations would catastrophically depart from their corresponding data.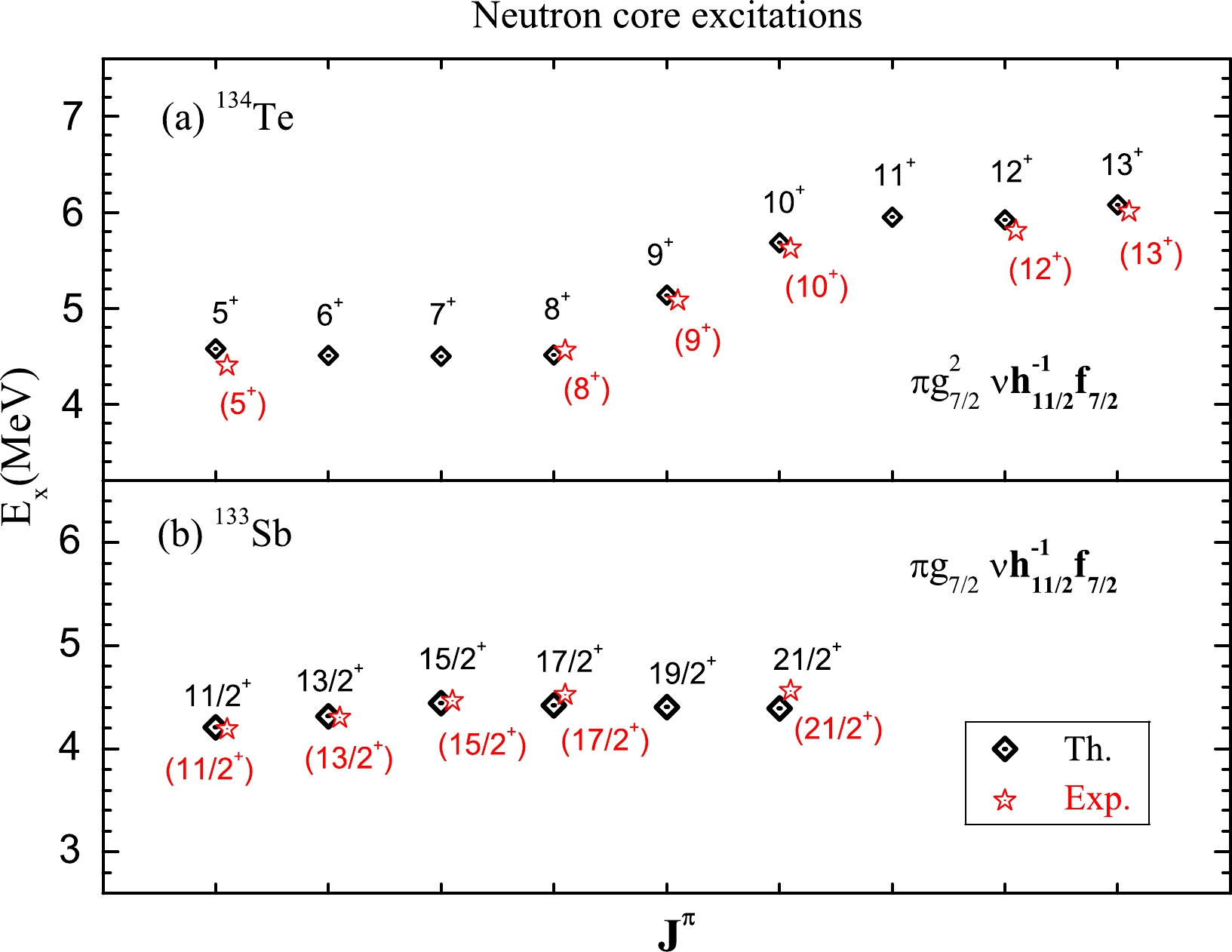

Figure 1

Figure 1.  (color online) Neutron core excitations in $^{134}$Te and $^{133}$Sb nuclei. Corresponding data are from Ref. [32].

DownLoad: Full-Size Img PowerPoint

Table 1

Table 1.  17$^+$ states in $^{132}$Te with main configurations. The data are from Ref. [14].

$^{132}$Te	$E_x$	(MeV)	Config.
$J^{\pi}$	Th.	Exp.	No.	P(\%)
(17$^+$)	8.189	6.166	1. $\pi g^2_{7/2}\nu h^{-3}_{11/2} f_{7/2}$	88
	8.779		2. $\pi g_{7/2} h_{11/2} \nu g^{-1}_{7/2} h^{-1}_{11/2}$	76
	9.125		3. $\pi g_{7/2} h_{11/2} \nu d^{-1}_{5/2} h^{-1}_{11/2}$	72
	9.314		4. $\pi h^2_{11/2} \nu h^{-2}_{11/2}$	93

DownLoad: CSV

Show Table

After excluding neutron core excitation, we focus on the level at 8.779 MeV coupled by config.2 $\pi g_{7/2} h_{11/2} \nu g^{-1}_{7/2} h^{-1}_{11/2}$. This level can be affected by these monopole terms from this configuration, i.e., Mc($\pi g_{7/2}$,$\pi h_{11/2}$), Mc($\nu g_{7/2}$,$\nu h_{11/2}$), and Mc($\pi g_{7/2}$,$\nu h_{11/2}$). The level at 8.779 MeV is abandoned, since the biggest difference is only 0.227 MeV in level 3$^-$ of $^{132}$Sb (Fig. 2). For the 17$^+$ level at 9.314 MeV, it has a new monopole term of Mc($\pi h_{11/2} \nu h_{11/2}$). This monopole term has almost no effects in levels 17$^+$ coupled by config.1, 2, and 3. $(\kappa = 0.1 \sim 0.9~\rm MeV)$, while the level 17$^+$ at 9.314 MeV drops to 8.3 MeV (Fig. 3). The level at 9.134 MeV is excluded too, because all 17$^+$ levels are increased sharply when the strength is $\kappa > 0.9~\rm MeV$. For the last one coupled by config.3, the suitable monopole term $Mc(\nu d_{5/2},\nu h_{11/2})$ is turned up from this configuration. Its monopole effects are investigated in the states of $^{132}$Te from 0$^+$ to 17$^+$ by adding $Mc(\nu d_{5/2},\nu h_{11/2})$ = –2.6 MeV. As shown in Fig. 4, the level 17$^+$ is reduced to 6.311 MeV, but the values of the 5$^-$ and 7$^-$ levels become far from the corresponding data.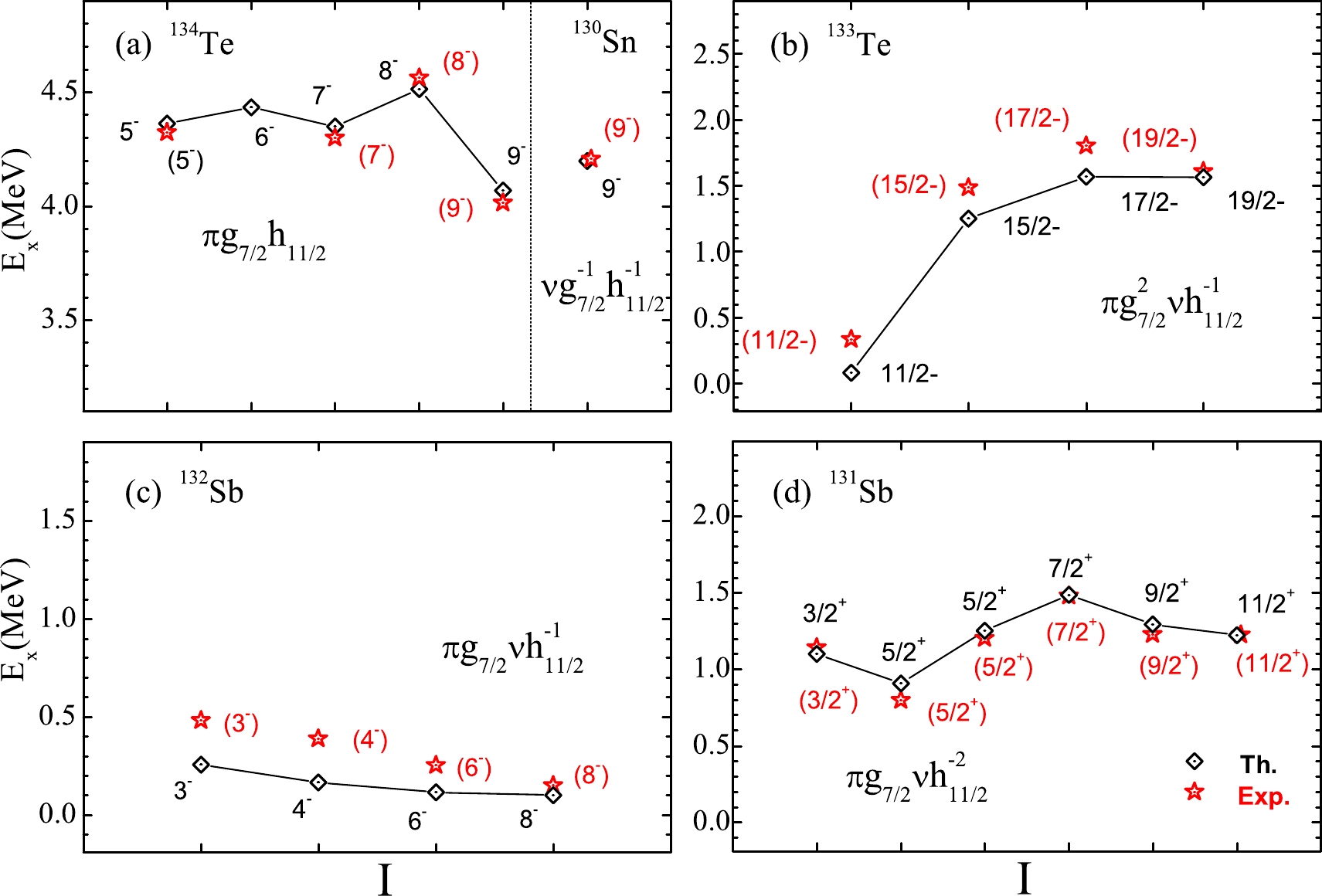

Figure 2

Figure 2.  (color online) Levels produced by particular configurations in $^{134}$Te, $^{133}$Te, $^{132}$Sb, $^{131}$Sb, and $^{130}$Sn nuclei, in comparison with given data [32].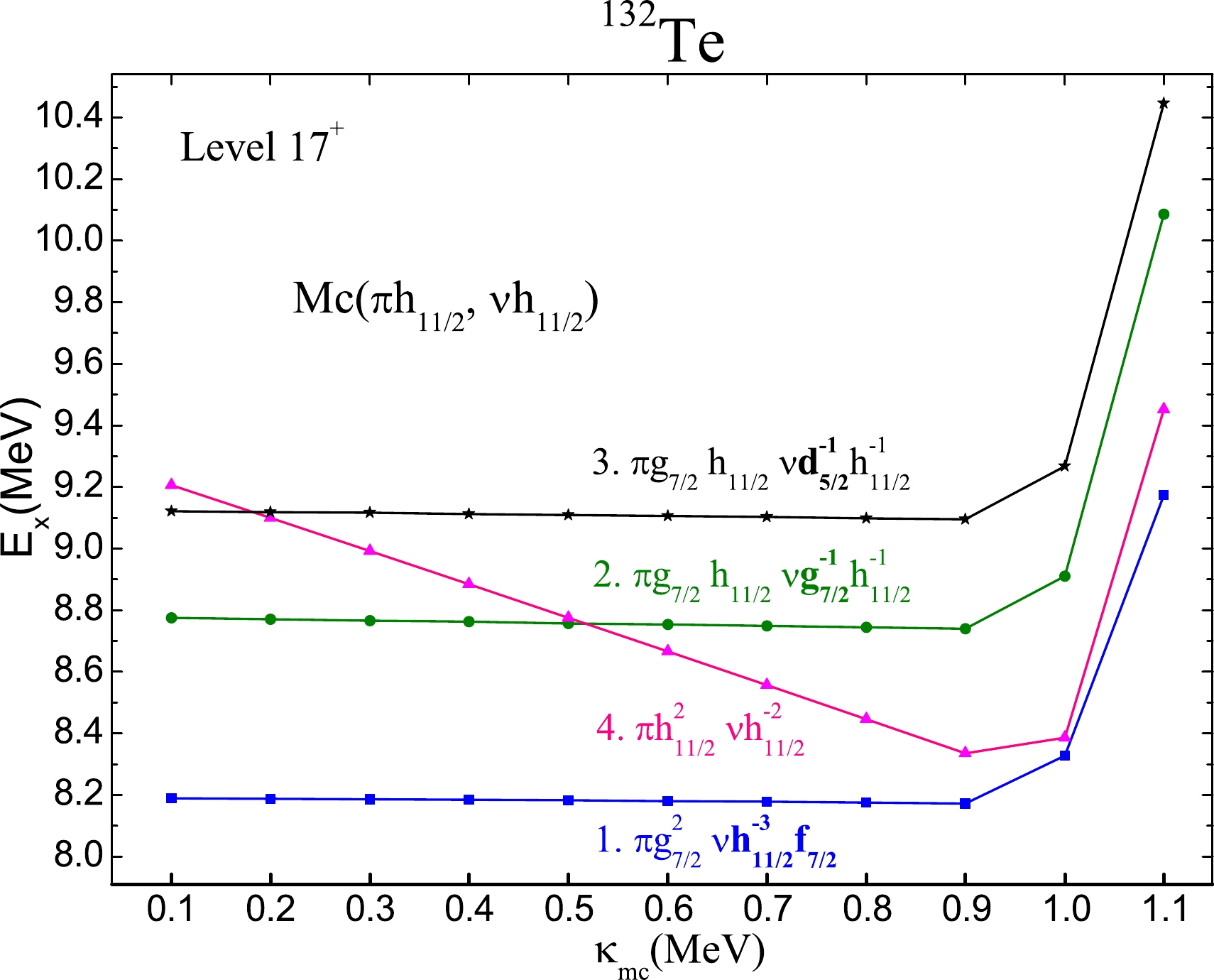

DownLoad: Full-Size Img PowerPoint

Figure 3

Figure 3.  (color online) Monopole effects of Mc($\pi h_{11/2} \nu h_{11/2}$) in level $17^+$ of $^{132}$Te.

DownLoad: Full-Size Img PowerPoint

Figure 4

Figure 4.  (color online) Monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$ in $^{132}$Te. Data marked with stars are from Ref. [32].

DownLoad: Full-Size Img PowerPoint

As shown above, the monopole interaction alone cannot solve the present puzzle, and we focus on the quadrupole-quadrupole force between the proton and neutron ($QQ_{\pi,\nu}$).

As shown in Fig. 5, the value of the$QQ_{\pi,\nu}$ force equals the quadruple-quadruple force strength divided by $[1/A(^{132}Sn)]^{5/3}$. The level 17$^+$ coupled by configuration $\pi g_{7/2}h_{11/2} \nu h^{-1}_{11/2} d^{-1}_{5/2}$ is reduced by 2.121 MeV when the QQ force changes from 300 to –450. The lowest value is 7.107 MeV, which occurs when $QQ_{\pi \nu}$ = –350. It seems the $QQ_{\pi,\nu}$ force provides a new method to explain the large difference in level 17$^+$. As shown in Fig. 6, the datum (17$^+$) is reproduced very well with $QQ_{\pi \nu}$ = –350 and $Mc(\nu d_{5/2}, \nu h_{11/2}) = -0.8~\rm MeV$. Furthermore, the values of 5$^-$ and 7$^-$ levels are close to data (5)$^-$ and (7)$^-$. The levels 13$^-$ and 14$^-$ drop by approximately 1 MeV with $QQ_{\pi,\nu}$ correction $(Q_c)$. The levels 3$^-$ and 14$^-$ are sensitive to the monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$. These levels are connected with datum 17$^+$ by the $QQ_{\pi,\nu}$ force and monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$. If they could be observed experimentally, this would be evidence confirming the datum (17$^+$).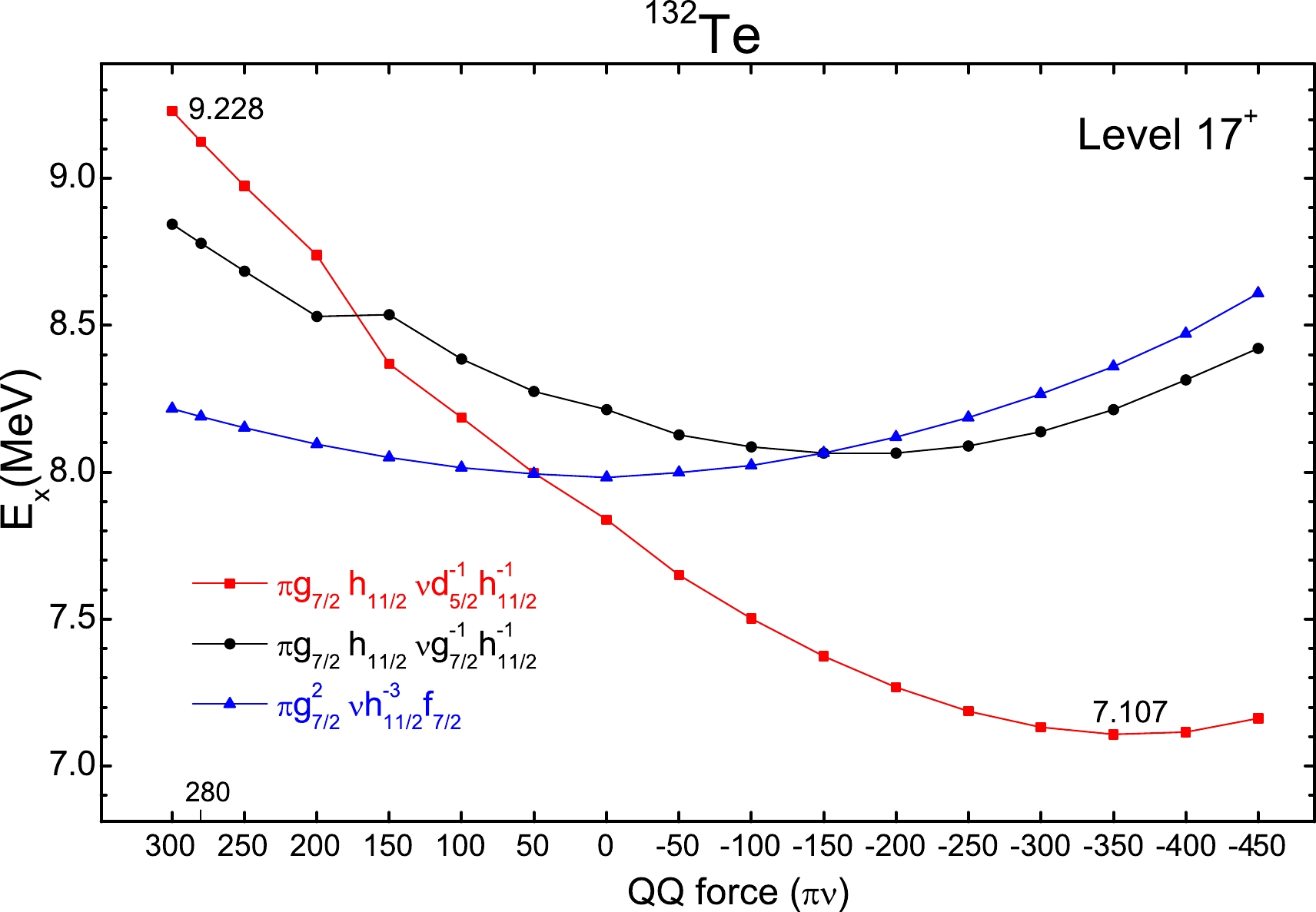

Figure 5

Figure 5.  (color online) Effects of the quadruple-quadruple force between the proton and neutron in level 17$^+$ of $^{132}$Te.

DownLoad: Full-Size Img PowerPoint

Figure 6

Figure 6.  (color online) $QQ_{\pi,\nu}$ force and monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$ in $^{132}$Te. Data marked with stars are from Refs. [14, 32].

DownLoad: Full-Size Img PowerPoint

For determining the $QQ_{\pi,\nu}$ correction and monopole term $Mc(\nu d_{5/2},\nu h_{11/2})$, an alternative of lack data in $^{132}$Te is to study the $QQ_{\pi,\nu}$ correction and monopole effects in other nuclei nearby. The monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$ also exist in $^{130}$Sn and $^{131}$Sb. In $^{130}$Sn, the configuration $\nu h^{-1}_{11/2} d^{-1}_{5/2}$ produces levels from 3$^-$ to 8$^-$. With the $Mc(\nu d_{5/2},\nu h_{11/2})$, the configuration percentages have almost no change in levels 3$^-$, 7$^-$, and 8$^-$, while the percentage of level 5$^-$ (6$^-$) drops from 57$\%$ (49$\%$) to 52$\%$ (39$\%$). Level 3$^-$ is the first state of $J^{\pi} = 3^-$, and its energy decreases by 1.01 MeV when Mc is added. Here, the $QQ_{\pi,\nu}$ force has no effect on one-shell closed nuclei. If this state could be observed near 2.199 MeV, this would be evidence for considering monopole correction in particle-hole nuclei near $^{132}$Sn. The same applies to level 8$^-$ as the first state of $J^{\pi} = 8^-$.

In $^{131}$Sb, levels from 1/2$^-$ to 21/2$^-$ have a main configuration of $\pi g_{7/2} \nu h^{-1}_{11/2}$. As shown in Fig. 7(b), the $QQ_{\pi,\nu}$ correction has obvious effects on levels 1/2$^-$, 3/2$^-$, 5/2$^-$, 21/2$^-$, and 23/2$^-$, while monopole correction Mc has almost no effects except in the case of level 23/2$^-$. The lowest energy level of $J^{\pi} = 23/2^-$ drops to 2.570 MeV with Qc and Mc. We are very interested in whether the high-spin state 23/2$^-$ can be observed experimentally. This 23/2$^-$ level can be used to determine the necessity of $QQ_{\pi,\nu}$ correction in the particle-hole nuclei region and finally explain the large difference in level (17$^+$) of $^{132}$Te.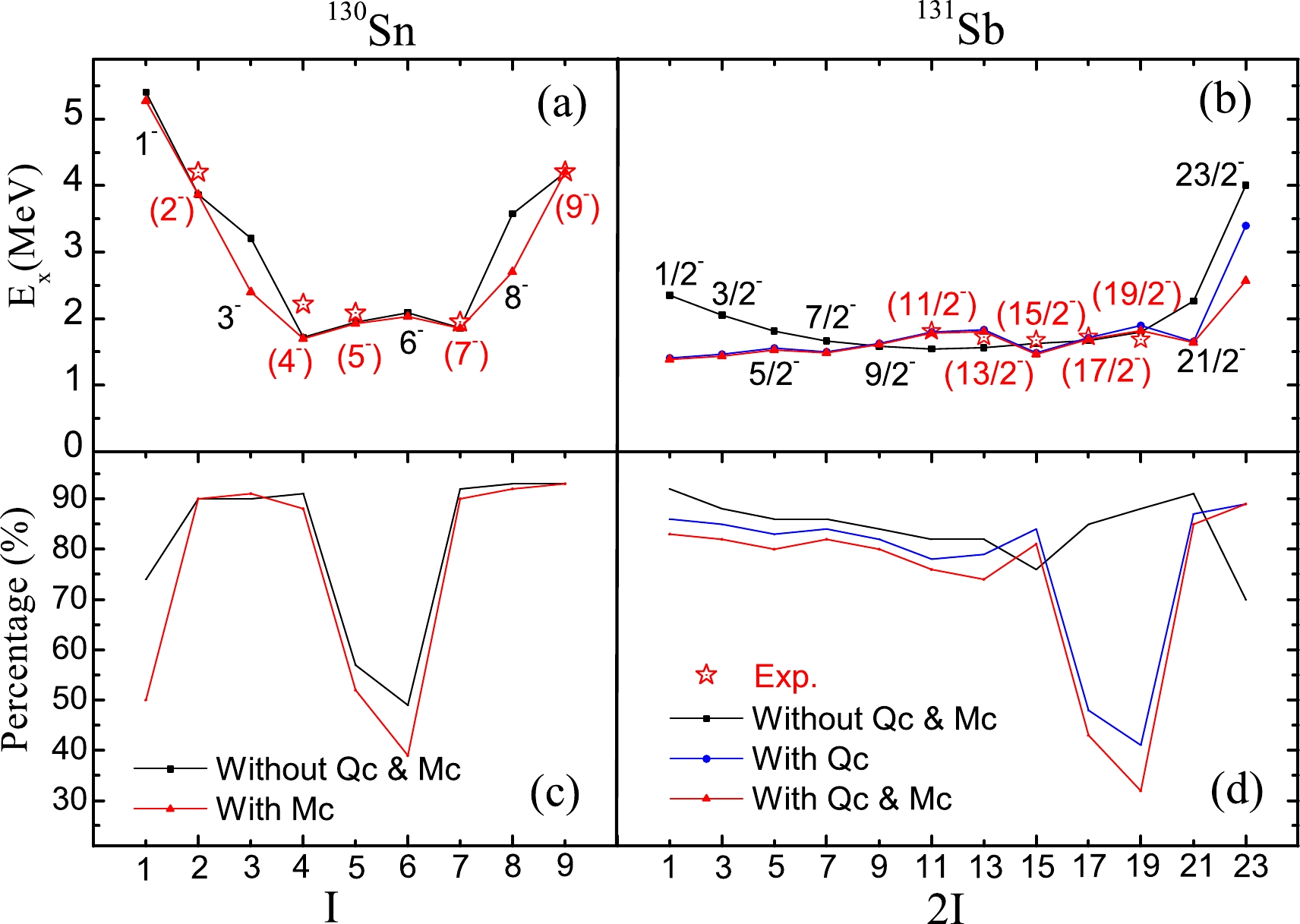

Figure 7

Figure 7.  (color online) $QQ_{\pi,\nu}$ force and monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$ in $^{130}$Sn and $^{131}$Sb. Data marked with stars are from Ref. [32].

DownLoad: Full-Size Img PowerPoint

IV. CONCLUSION

• I. INTRODUCTION
• II. HAMILTONIAN AND MONOPOLE EFFECTS
• III. HIGH-SPIN LEVELS
• IV. CONCLUSION

The monopole effects and high-spin levels in $^{132-134}$Te, $^{131-133}$Sb, and $^{130}$Sn are investigated. The datum 6.166 MeV of level 17$^+$ in $^{132}$Te is excluded from configurations $\pi g_{7/2} h_{11/2} \nu g^{-1}_{7/2} h^{-1}_{11/2}$ or $\pi h^2_{11/2} \nu h^{-2}_{11/2}$. The present work suggests the datum (17$^+$) coupled by configuration $\pi g_{7/2} h_{11/2} \nu d^{-1}_{5/2} h^{-1}_{11/2}$. Several levels are connected with state (17$^+$) by monopole effects of $Mc(\nu d_{5/2},\nu h_{11/2})$ and the quadruple-quadruple force between the proton and neutron, i.e., 3$^-$ (8$^-$) in $^{130}$Sn, 14$^-$ in $^{132}$Te, and 23/2$^-$ in $^{131}$Sb. If these states could be observed at lower energies, the lower state (17$^+$) of $^{132}$Te would be explained with quadruple-quadruple correction between the proton and neutron and the increasing strength of the monopole interaction between neutron orbits $d_{5/2}$ and $h_{11/2}$.

References