Collisional Penrose process of 4D rotational Einstein-Gauss-Bonnet black holes

General relativity (GR) is the most successful theory to explain the relationship between geometry and matter. It has predicted some phenomena that would have been unthinkable in the past, such as supernova outbursts and gravitational waves [1]. However, GR still has some problems that cannot be explained, such as the geodesic incompleteness that results from singularities [2]. Therefore, quantum theories of gravity have been developed, and hence the Gauss-Bonnet (GB) term is naturally raised from the perturbation approximation of quantum theories [3, 4].

The action of gravity with the Gauss-Bonnet correction reads

with

where R is the Ricci scalar curvature, and $ \alpha $ is a dimensionless coupling constant of D-dimensional EGB gravity with $ D>4 $ .

However, 4-dimensional GB gravity is hard to get because the GB term is a purely topological invariant according to the well-known Lovelock theory, and the theory indicates that the GB term only exists in spacetime dimension $ D>4 $ [5, 6]. For string-generated gravity models, Boulware and Deser [7] gave the first spherically symmetric static black hole solution. Although the GB Lagrangian ${\cal{L}}_{\rm GB}$ does not play an additional role in gravitational dynamics, at the quantum level, Tomozawa [8] proved that a meaningful impact of the GB term would be created from the regularization procedure.

Subsequently, using the dimensional limit $ D \rightarrow 4 $ , Cognolo et al. [9] obtained the entropic corrected static spherically symmetric metric in 4-dimensional spacetime. Recently, Glavan and Lin put forward a new modified theory called 4D Einstein-Gauss-Bonnet (EGB) gravity [10]. The theory bypasses the Lovelock theorem by using an ingenious coupling constant $ \tilde{\alpha} \rightarrow \alpha/(D-4) $ . 4D EGB gravity has some contributions to gravitational dynamics and its own fascinating characteristics. For example, it forecasts a new spherically symmetric static black hole solution,

where $ f(r) = r^2+{r^4}/{(2 \alpha) } \left(1 \pm \sqrt{{8 \alpha M}/{r^3}+1}\right) $ , $ \alpha $ is the EGB coupling parameter and M is the black hole mass.

Others have studied various aspects of this theory of gravity [11-22].

There have been doubts about whether the theory makes sense in general in its use of this ingenious coupling constant [23-27]. Fortunately, however, it soon became clear that this 4D EGB gravity guarantees well-defined extensions [28-31] and most importantly, the spherically symmetric static black hole solution (4) is still a vacuum solution in the new theory.

On the other hand, in 2009, Bañados, Silk and West (BSW) came up with a new idea that rotating black holes can accelerate non-spinning particles as particle accelerators [32]. When we take the extreme Kerr black hole as an example, the center-of-mass energy of collisional particles can be infinitely high [32]. After that, the BSW mechanism was extended to spinning particles [33-43]. Besides, we know that spinning particles are no longer moving along geodesics in curved spacetime and the motion of spinning test particles can be described by modified equations [44-48]. In Ref. [49], some universal conclusions have been obtained on the BSW mechanism.

Along with this idea, some researchers have reconstructed the Penrose process from the BSW process [50-52]. The energy extraction efficiencies of these collision processes are much higher than the traditional Penrose process. For instance, Schnittman found that the energy extraction efficiency comes up to 13.92 for Compton scattering near the event horizon [52]. Similarly, in 2015, Berti considered the case where one of the original colliding particles falls from infinity while another particle is created in the ergosphere [53]. This process was quickly generalized to spinning particles [54]. To date, the collision Penrose process has been investigated under different curved backgrounds with different types of particle [54-60].

Hence, the purpose of this paper is to study the collision Penrose process of four-dimensional rotating black holes in EGB theory and look at how different parameters affect energy extraction. Moreover, when the coupling constant $ \alpha\neq0 $ , the corresponding rotational black hole is an extension of the Kerr solution [61, 62]. In Refs. [62-65], novel and attractive results have been found for 4D EGB metrics with coupling $ \alpha<0 $ . Hence, the study of the corresponding Penrose process may give us a new and attractive result and thus in turn provide a possible experiment to distinguish EGB gravity from general relativity in principle.

The paper is structured as follows. In Sec. II, we obtain the motion of spinning particles for a rotational four-dimensional EGB black hole. In the next section, constraints on the orbits, including radial constraints and time-like constraints, are investigated. In Sec. IV, we get the solution of energy extraction efficiency with different initial values. We give our conclusions in the final section. We adopt geometrical units $ ({c} = {G} = 1) $ to calculate the equations throughout the paper.

In order to obtain the equations of motion of spinning particles, we assume that the collision particles are test particles, and particles have no back-reaction on the spacetime background.

We consider an axially symmetric and stationary spacetime. Hence, using the modified procedure without complexification [66] of the Newman-Jains (NJ) algorithm [67] to generate the rotational solutions of 4D EGB from the static spherically symmetric solution of Eq. (4), the resulting 4-dimensional line element of a rotating black hole, in Boyer-Lindquist coordinates $ (t,r,\theta,\phi) $ , reads as [61, 62]

with

where a is the angular momentum and M is the mass of the EGB black hole. When $ \alpha \rightarrow 0 $ , the rotational EGB black hole will go back to a Kerr black hole. It should be noted that this rotational solution is no longer a vacuum solution which contrasts with GR, as the Kerr solution can also be obtained from the Schwarzschild solution via the NJ algorithm, and both black holes are vacuum solutions. This is common in modified gravity theories if one uses the NJ algorithm to generate the rotational black holes from static spherically symmetric solutions which may introduce some additional matter fields to the spacetime [66, 68-71].

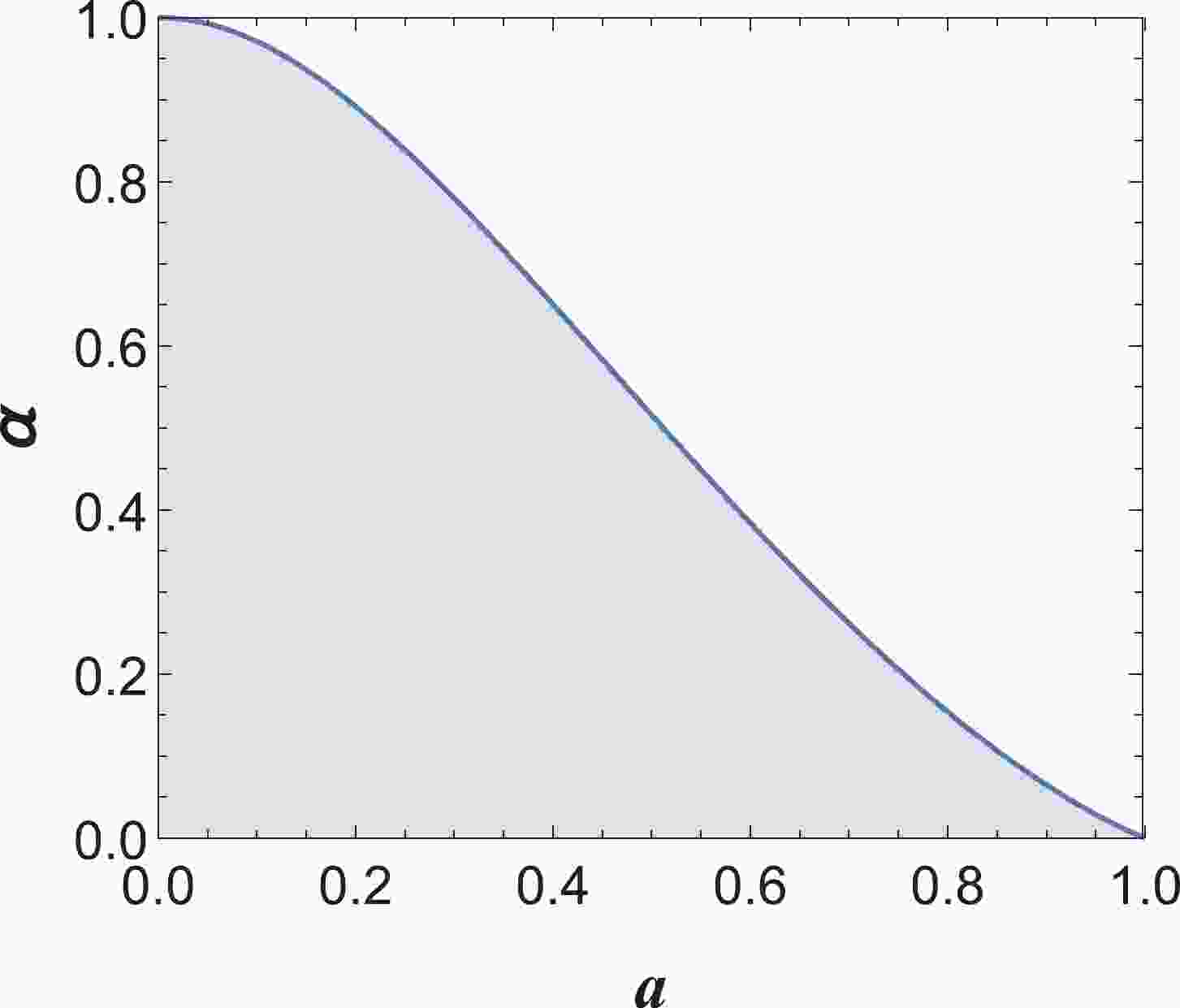
In this paper, we consider the case of $ \alpha>0 $ as well as the case $ \alpha<0 $ . The horizon of the EGB black hole can be obtained by solving the equation $ \Delta = 0 $ . We will pay attention to the event horizon $ r_h $ , which is located at the largest root of the above equation $ \Delta = 0 $ .

For instance, when $ \alpha>0 $ , the shaded part in Fig. 1 indicates the existence of an event horizon. The black hole has only one event horizon solution when a and $ \alpha $ are on the shadow boundary (blue line). When a and $ \alpha $ are inside the shadow, the black hole has two event horizons. This means that the blue line represents the relationship between a and $ \alpha $ for the extreme black hole. Similar relations for $ \alpha<0 $ can be found in Fig. 1 of Ref. [62].

Figure 1.  (color online) Relation of a and $ \alpha $ for the existence of horizons in EGB gravity.

The equations of motion of a spinning particle can be described by the Mathission-Papapetrou-Dixon (MPD) [44-46] equations,

where $ S^{cd} $ is the antisymmetric spin tensor, $ R^{a}{}_{bcd} $ is the Riemann tensor, $ p^a $ is the four-momentum and $ v^{b} $ is the tangent vector along the trajectory. Note that Eq. (7) and Eq. (8) are not enough to describe the unique equation of motion for a particle, so we need some constraints:

With Eqs. (9) and (10), we can define the mass m and spin s of the particles. By posing one more supplementary condition [47],

then together with Eqs. (7) and (8) we can uniquely determine the motion of the particles.

Finally, we can normalize the affine parameter $ \tau $ such that $ p^a v_a = -m $ . One can obtain the difference between the 4-momentum $ p^a $ and 4-velocity $ v^a $ as

For later convenience, we use a tetrad basis to simplify the calculations. The tetrad basis reads

For spinning particles, the conserved quantities in EGB spacetime can be given as

where $ \xi_a $ is the Killing vectors. Note that the Killing vectors in EGB spacetime are $ \xi^a = \left(\partial/\partial t\right)^a $ and $ \phi^a = \left(\partial/\partial\phi\right)^a $ .

The exact expression of the spin tensor $ S^{(a)(b)} $ has yet to be found. So, we define a spin vector $ s^{(a)} $ ,

where the completely anti-symmetric tensor $ \varepsilon_{(a)(b)(c)(d)} $ has the convention $ \varepsilon_{(0)(1)(2)(3)} = 1 $ . For the sake of simplicity, we give priority to the motion in the equatorial plane ( $ \theta = \pi /2 $ ) [72]. Hence, the antisymmetric spin tensor $ S^{(a)(b)} $ can be re-described as

This implies that particle spin is parallel to the angular momentum of the EGB black hole with $ s>0 $ , and anti-parallel with $ s<0 $ .

Equations (14) and (16) give

where E is the energy and J the angular momentum of the particle. Thus, from the equations above, we obtain

where

The missing term $ p^{(1)} $ can be obtained by the normalization condition of the 4-momentum as

where m is the mass of the particle.

Furthermore, making use of the MPD equations, we get the difference between the 4-momentum and 4-velocity as

where

where the expression of $ \Pi_1 $ is complicated and is given in the Appendix. $ u^{(a)} $ is the specific momentum of a massive particle,

Finally, the detailed expressions for the motion of a spinning particle are:

where the detailed expression of R is given in the Appendix.

Without loss of generality, we use dimensionless parameters instead of the original parameters, as follows:

For simplicity of expression, we remove the $\widetilde{\hphantom{0}}$ on the dimensionless parameters, so e.g. J means $ \tilde{J} $ , in the remainder of the paper.

In the following, we assume that the particle collisions occur near the event horizon of the black hole. This requires that the radial motion expression ${\rm d}r/{\rm d}\tau$ (Eq. (31)) has a real solution near the the event horizon. This implies that $ R\geqslant 0 $ must be satisfied at $ r>r_h $ . From Eqs. (19), (20), (23) and (29), we know that R in Eq. (A1) equals $ -1-(u^{(3)})^2+(u^{(0)})^2 $ . So we further simplify and redescribe R as

where

The roots of the equation $ R = 0 $ then read:

The positive ( $ J_+ $ ) and negative ( $ J_- $ ) solutions for radial motion are plotted in Fig. 2.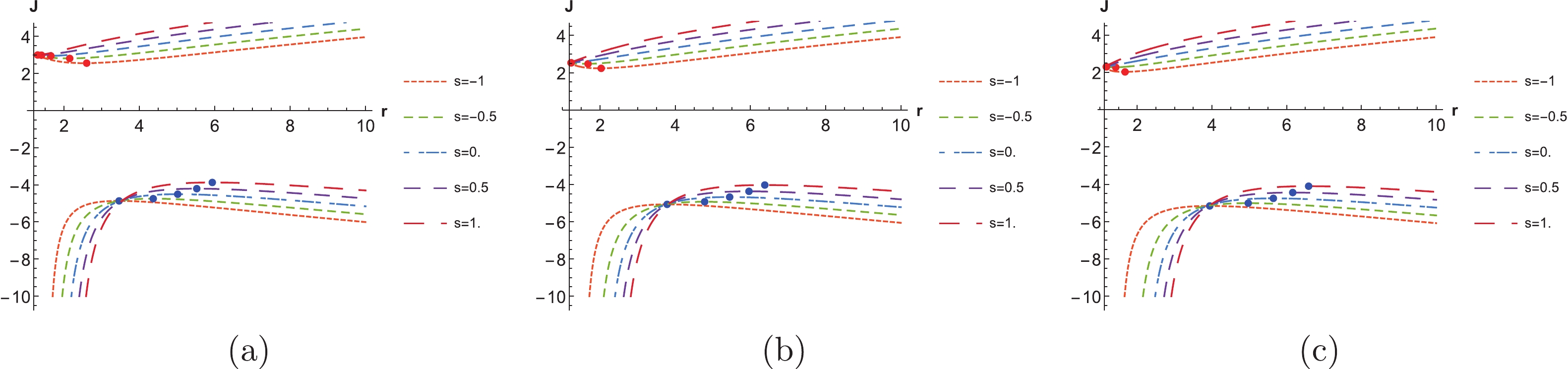

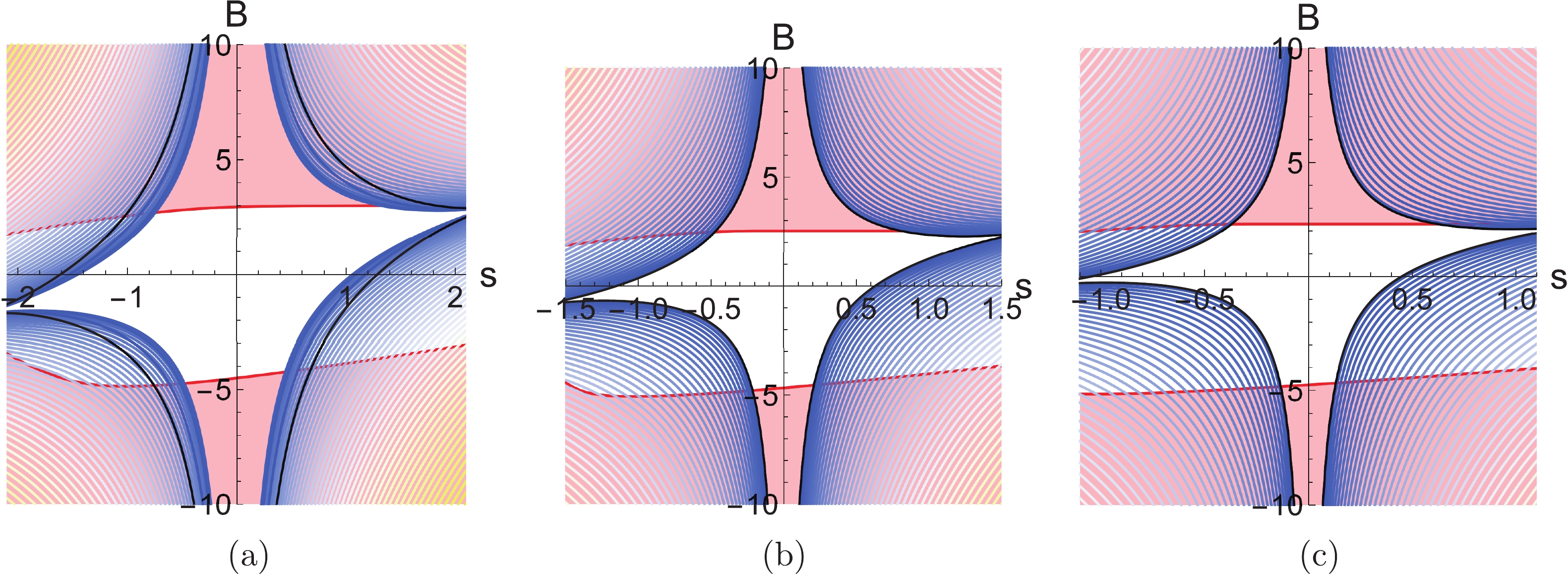
Figure 2.  (color online) Constraints on J ( $ B = J $ ) with $ E = 1 $ and (a) $ a = 0.6 $ , (b) $ a = 0.8 $ and (c) $ a = 0.9 $ , for different values of s in extreme spacetime.

In Fig. 2, we give the upper and lower bounds of J with red and blue points respectively. Only when the particle parameter is located between the red and blue points can outgoing particles move from the horizon to infinity, or ingoing particles fall into the neighborhood of the event horizon from infinity.

It is important to note that we can find an estimation of the critical value $ B_{c+} \approx -Y_2/Y_1 $ of particle 3 from Eq. (42) when $ E_3 $ is large. For example, $ B_{c+}\approx 2.3 $ with $ a \approx 0.9 $ for extreme rotational EGB black holes.

More importantly, the 4-velocity of a massive particle must satisfy the timelike condition,

Combining Eqs. (24), (25), (26) and (29), we have

By eliminating the parameters $ u^{(0)} $ and $ u^{(1)} $ , we have

Note that $ {\Pi_1}^2>0 $ , and combining Eqs. (20) and (29), we find the condition

In Eq. (46), if $ \Pi_3(\Pi_3-2 \Pi_2) \leqslant 0 $ , timelike constraints will naturally be satisfied. So, if $ \Pi_3(\Pi_3-2 \Pi_2)>0 $ , the solution of this equation gives

where

Figure 3 shows the permitted scope for the spin s and energy E when $ a = 0.8 $ and $ B = 2 $ at $ r = r_h $ for an extreme black hole. From Fig. 3, because of symmetry, we only have to take care of the case where the energy is greater than zero ( $ E>0 $ ). Next, if particles free-fall from infinity, with energy $ E = 1 $ , combining with the condition (47), we have the allowable range of spin s as $ s_{\min} < s < s_{\max} $ for a given value of B and r. To further consider the parameters B and r, Eq. (47) can be redescribed as

Figure 3.  (color online) Relation between E and s.

The particle is located in the neighborhood of the event horizon $ r = r_h $ and the energy of the ingoing particle is normalized as $ E = 1 $ . The constraints on s and B are illustrated by the yellow shading in Fig. 4, and the result shows that the range of spin s is completely determined by the value of B.

Figure 4.  (color online) Constraint on s and B with $ E = 1 $ , with (a) $ a = 0.6 $ , (b) $ a = 0.8 $ , and (c) $ a = 0.9 $ , at $ r = r_h $ in the extreme case.

Combining the two constraints above, the admissible range of s and B is located in the white area in Fig. 5.

Figure 5.  (color online) Constraints on s and B with $ E = 1 $ , with (a) $ a = 0.6 $ , (b) $ a = 0.8 $ , and (c) $ a = 0.9 $ , in the extreme case.

Above, we obtained the equations of motion of spinning particles and the constraint conditions on the parameters s and $ B(B = J/E) $ . Now we move to the efficiency of energy extraction from extreme rotational EGB black holes. We consider that an ingoing particle, with 4-momentum $ p_1^\mu $ , collides with an outgoing particle which has 4-momenta $ p_2^\mu $ in the ergosphere of the extreme black hole. Then, we assume that particle 3 and particle 4 are going to infinity and falling into the black hole with 4-momenta $ p_3^\mu $ and $ p_4^\mu $ respectively. The collision parameters are chosen as $ -\sigma_1 = \sigma_2 = \sigma_3 = $ $ -\sigma_4 = 1 $ [56, 73]. In order to obtain the efficiency of energy extraction, we need the following conservation laws:

where Eqs. (50), (51), (52) and (53) give the conservation of the energy E, the angular momentum J, initial spins $ S^{ab} $ and radial components of 4-momentum $ p^{(1)} $ respectively. For the sake of simplification, we suppose that the masses of all the collision particles are equal to m. With this simplification, the conservation law of initial spins $ S^{ab} $ and radial components of 4-momentum $ p^{(1)} $ can be re-expressed as

We consider that the head-on collision of the two test particles takes place in the ergoshpere, with parameters $ s_3 = s_1 $ , $ s_2 = s_4 $ , $ E_1 = E_2 = 1 $ [56, 74].

Then Eq. (50) and Eq. (51) imply

where $ B_1 = J_1/E_1 = J_1 $ , $ B_2 = J_2/E_2 = J_2 $ and $ B_3 = J_3/E_3 $ . So Eq. (55) leads to the equation of parameters ( $ E_3, s_1, s_2, B_1, B_2, B_3, r, a, \alpha $ ). Note that the relation a and $ \alpha $ for the extreme black hole is given in Fig. 1. The detailed expressions for $ u_1^{(1)} $ , $ u_2^{(1)} $ , $ u_3^{(1)} $ and $ u_4^{(1)} $ are given in the Appendix.

Now we are ready to get the analytical solution for the energy of particle 3. First, we set $ s_1 = s_2 = s $ and $ B_1 = B_2 = B $ . Then $ {\left|{{u_1^{(1)}}}\right|} = {\left|{{u_2^{(1)}}}\right|} $ and $ s_1 = s_2 = s_3 = s_4 = s $ , which implies that the sum of radial momentum $ (u_1^{(1)}+ $ $ u_2^{(1)}) $ equals zero. Then Eq. (55) can be rephrased as $ R_3 = R_4 $ with $ Y_1 = Y_2 = Y_0 $ . The detailed expressions can be found in the Appendix. Solving the equation $ R_3 = R_4 $ gives

where

The energy contraction efficiency is defined as:

From the above equations, we know that the expression of $ E_3 $ is determined by five parameters ( $ E_3(s,B,B_3,r,a) $ ) in extreme rotational EGB black holes.

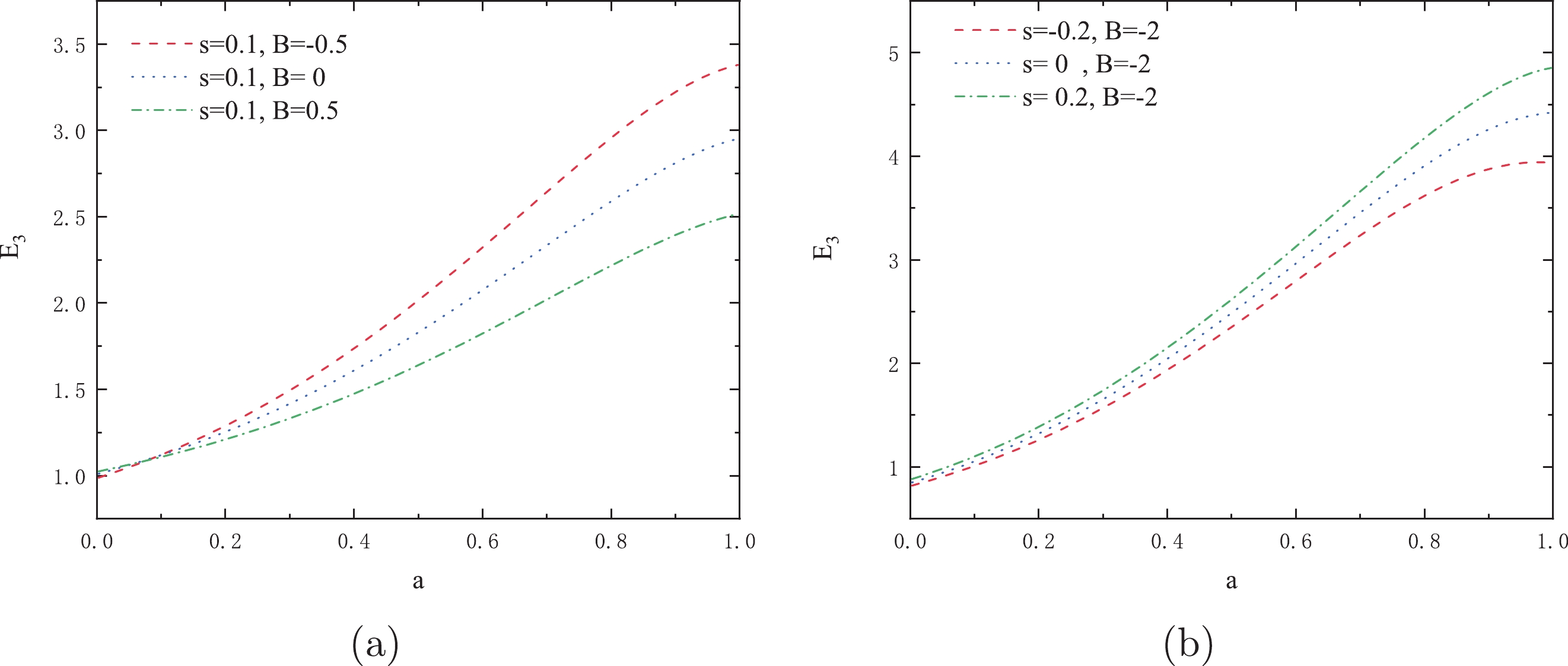
The relation of extracted energy $ E_3 $ of particle 3 with r is plotted in Fig. 6. $ E_3 $ increases as $ B_3 $ increases. The red point represents the maximum of $ E_3 $ for the given parameters.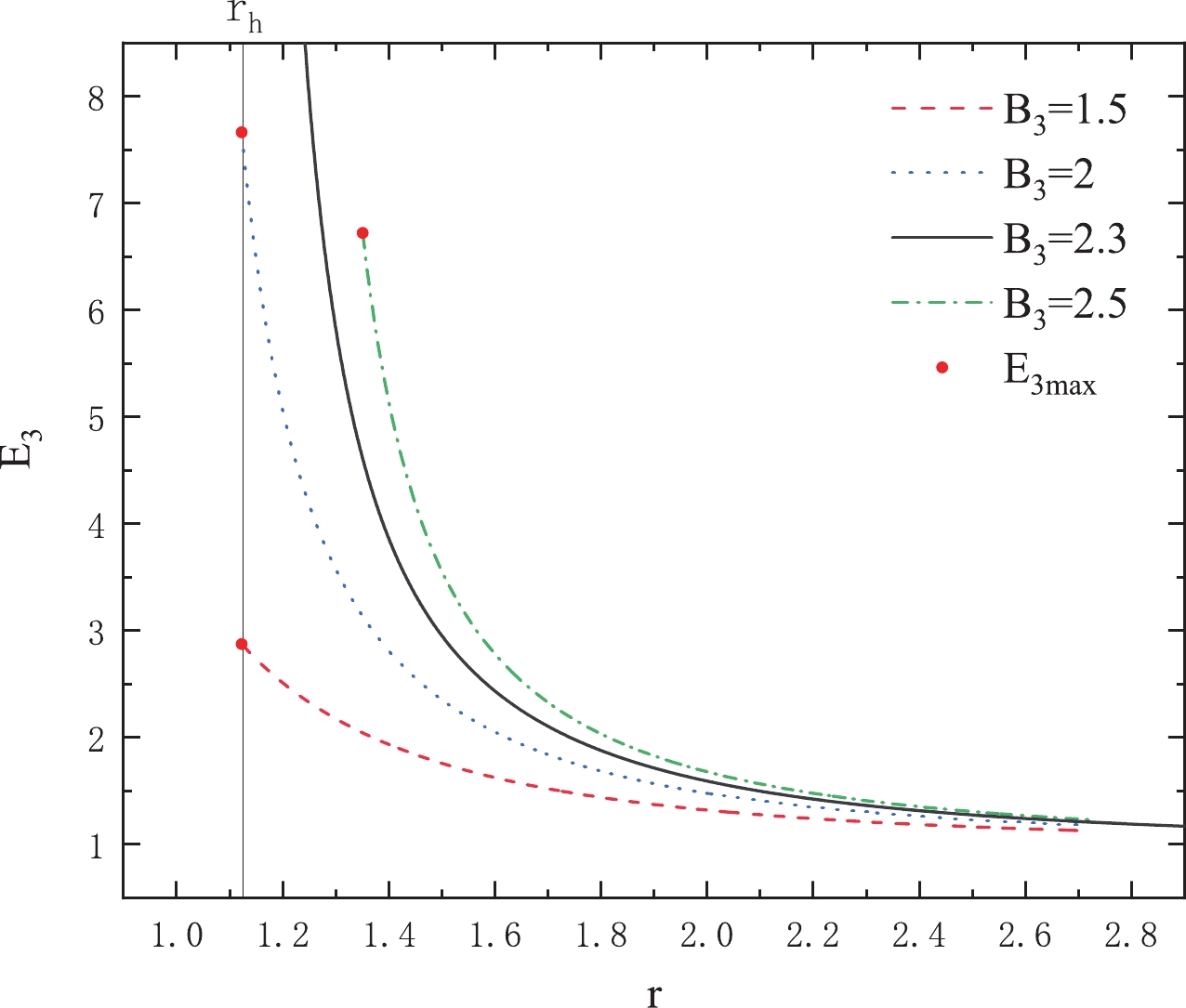

Figure 6.  (color online) Relation of r and $ E_3 $ with $ a = 0.9 $ , $ s = 0.1 $ , $ B = 0 $ and different values of $ B_3 $ .

The extracted energy $ E_3 $ of particle 3 with different values of spin s and B are plotted in Fig. 7. We find that for a fixed spin s, the extracted energy $ E_3 $ increases as B decreases, while for a fixed B, $ E_3 $ increases as spin s increases.

Figure 7.  (color online) Relation of a and $ E_3 $ with (a) $ s = 0.1 $ and (b) $ B = -2 $ . In both of the figures, $ B_3 = 2 $ and $ r = 1.4 $ .

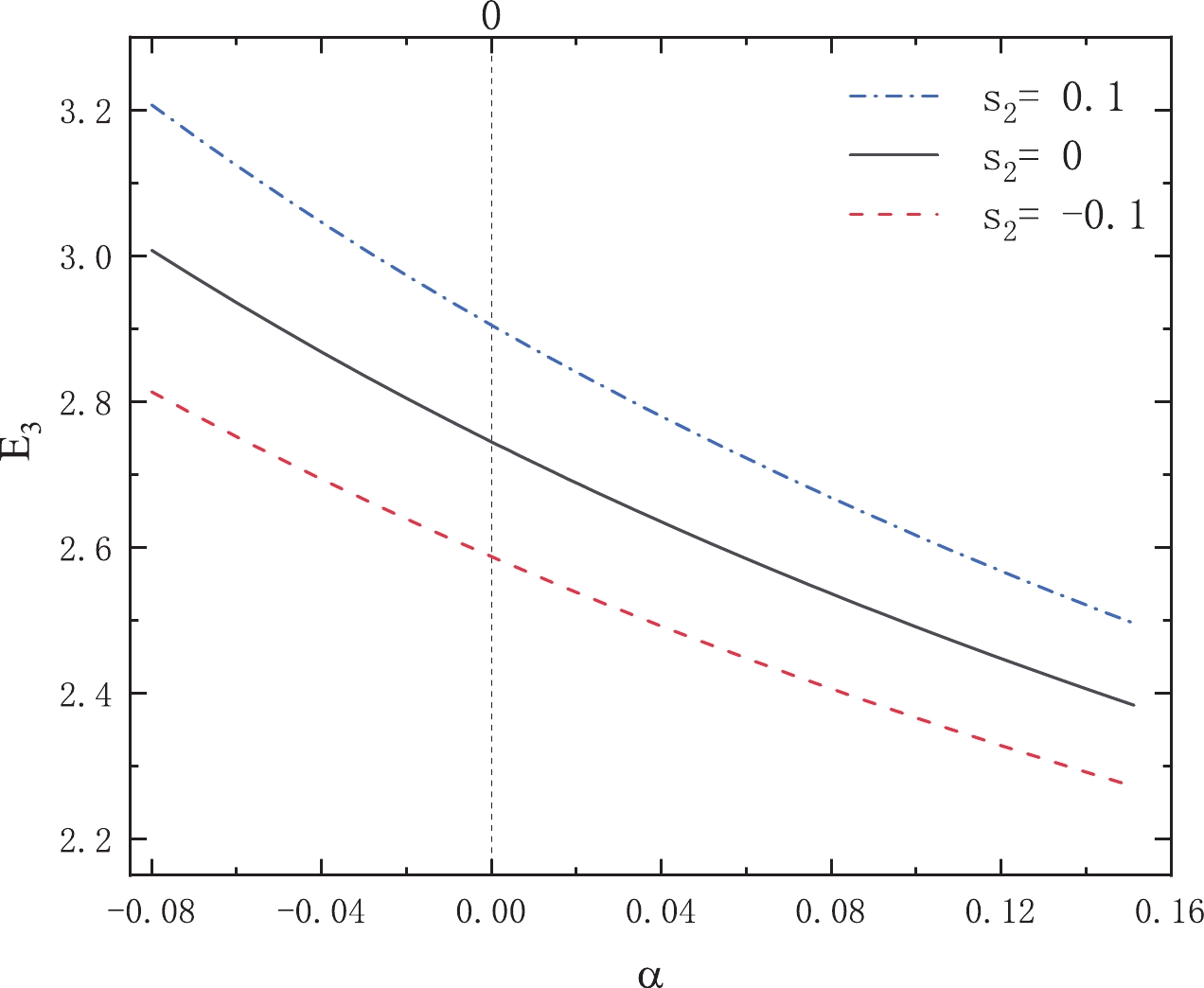
Now we come to study the case of $ \alpha<0 $ , and we consider the extracted energy $ E_3 $ of particle 3 with different values of EGB coupling parameter $ \alpha $ and particle spin s when other parameters are fixed. From Fig. 8, when $ \alpha<0 $ , we find that the extracted energy $ E_3 $ increases as EGB coupling parameter $ \alpha $ decreases.

Figure 8.  (color online) Relation of $ \alpha $ and $ E_3 $ with $ a = 0.8 $ , $ B = -2 $ , $ B_3 = 2.3 $ and $ r = 1.7 $ for different values of s.

In this paper, we have considered the collisional Penrose process of massive spinning particles in an extreme rotational EGB black hole background. By using the MPD equations, we obtained the equations of motion of spinning particles. Then we considered the constraint of the radial motion as well as the timelike condition and found the admissible range of s and B. We considered the hand-on collision with one particle falling from infinity and another particle created in the ergosphere. We calculated the energy extraction efficiency $ \eta = E_{3}/2 $ through the conservation laws during the collision. When the EGB coupling constant $ \alpha = 0 $ , the rotational EGB black hole reduces to the Kerr black hole, and therefore our results go back to the Kerr case, which has already been analysed in Refs. [54, 56].

For the parameter $ B = J/E $ , which is related to the particle's angular momentum, we find that the energy extraction efficiency increases monotonically with the decrease of parameter B for a given EGB coupling parameter $ \alpha $ . This means that the energy extraction efficiency can be higher when the total angular momentum of the initial ingoing particle is anti-parallel to the angular momentum of the black holes. Moreover, the efficiency $ \eta $ increases as the particle spin s grows. We also find that the energy extraction efficiency increases with the decrease of the EGB coupling parameter $ \alpha $ . This means that the EGB coupling parameter $ \alpha $ can enhance the efficiency of the collisional Penrose process when $ \alpha<0 $ compared to the case in general relativity.

The detailed expressions of R, $ u_1^{(1)} $ $ u_2^{(1)} $ $ u_3^{(1)} $ and $ u_4^{(1)} $

where